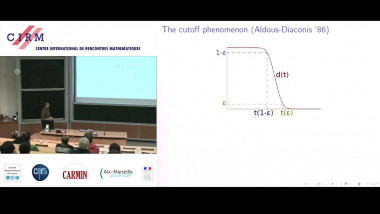
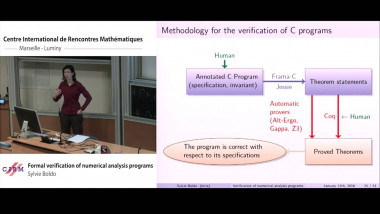
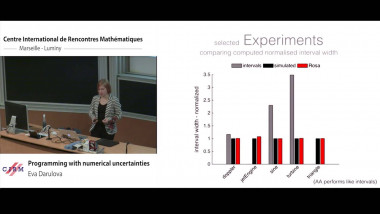
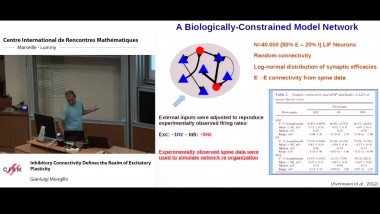
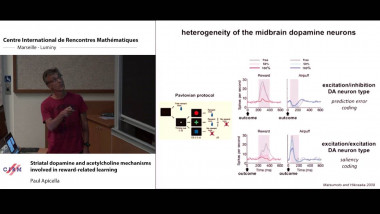
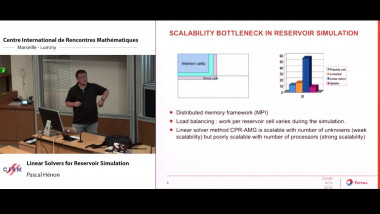
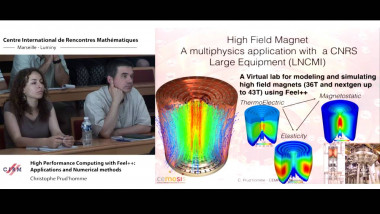
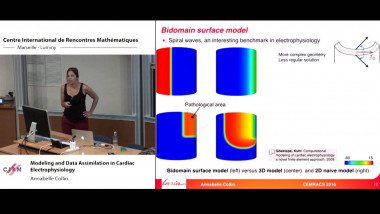
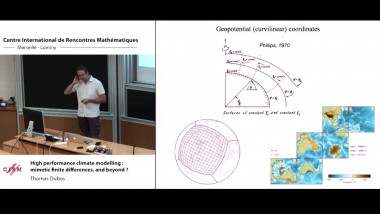
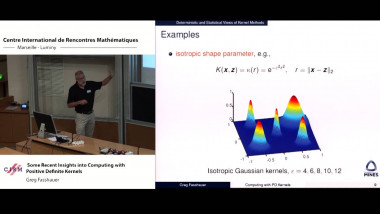
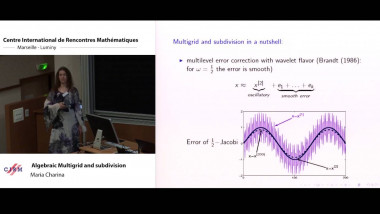
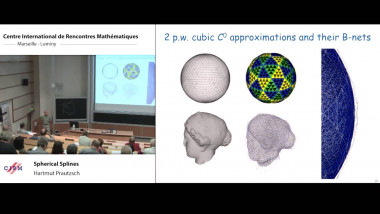
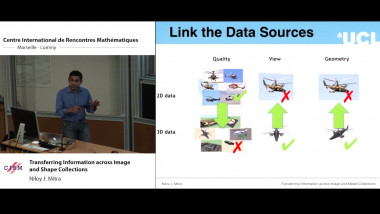
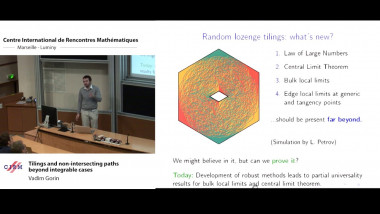
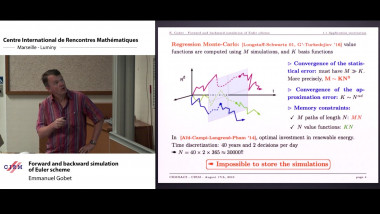
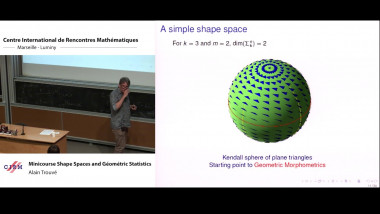
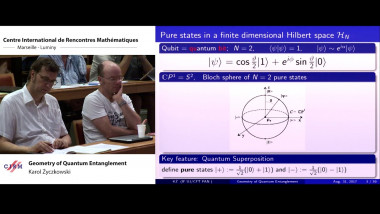
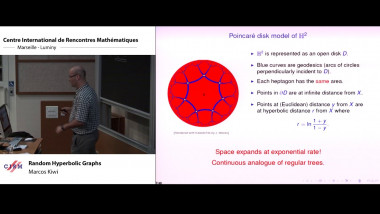
Bounded remainder sets for the discrete and continuous irrational rotation
Also appears in collection : Prime numbers and automatic sequences: determinism and randomness / Nombres premiers et suites automatiques : aléa et déterminisme
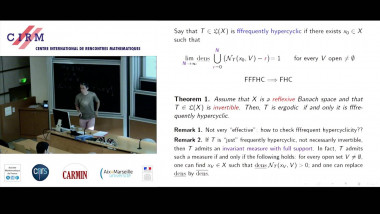
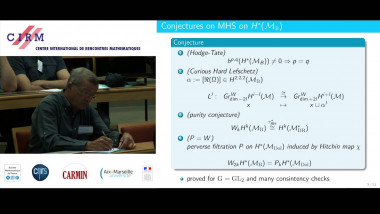
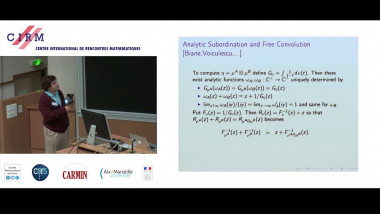
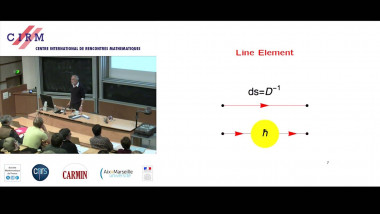
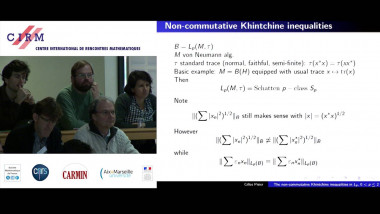
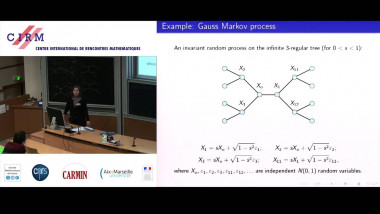
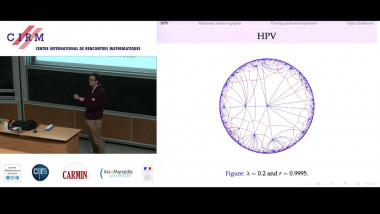
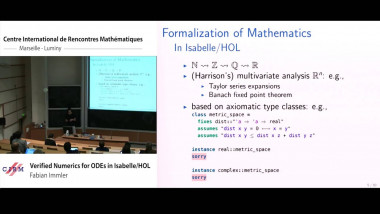
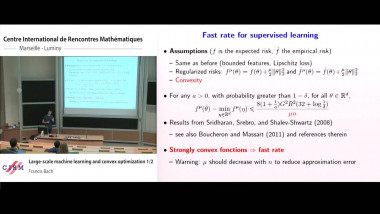
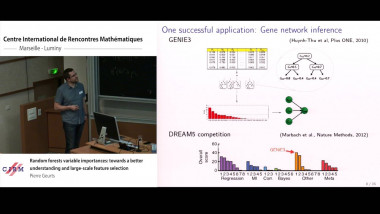
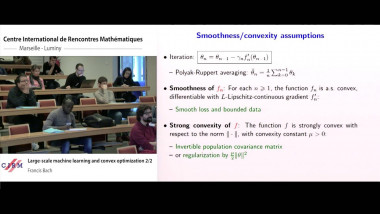
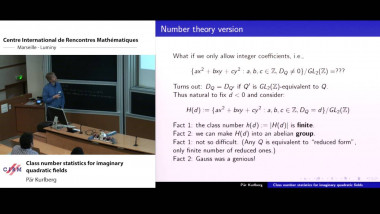
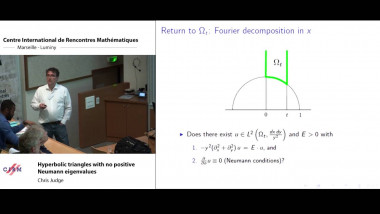
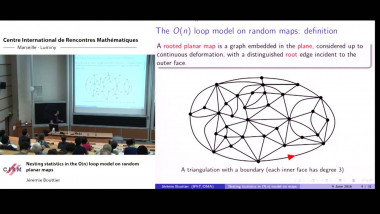
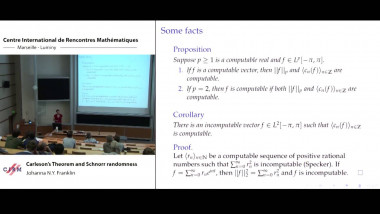
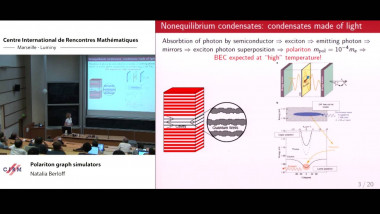
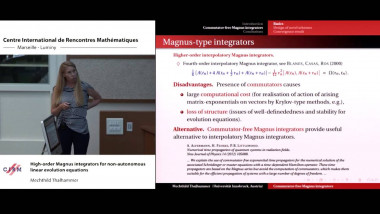
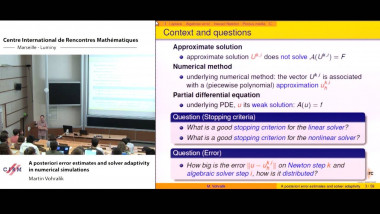
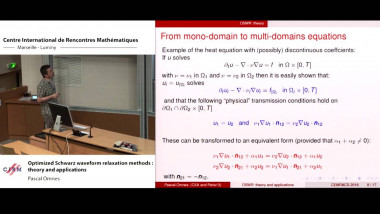
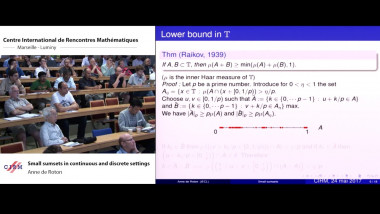
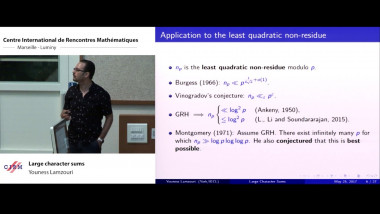
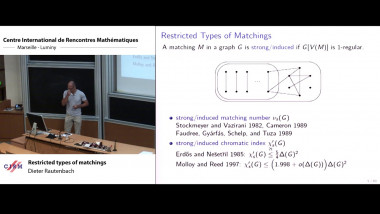
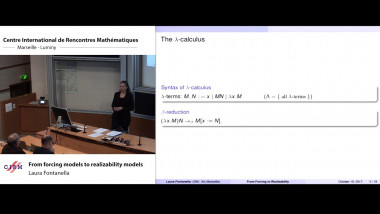
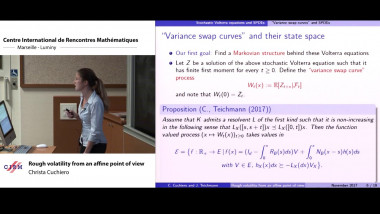
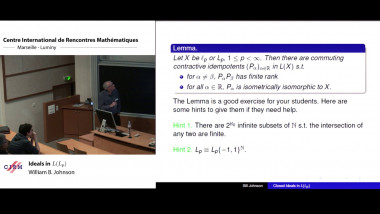
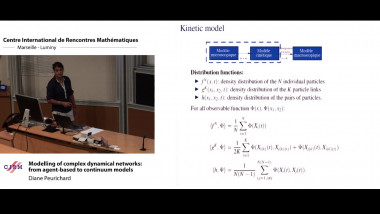
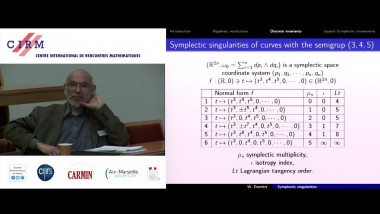
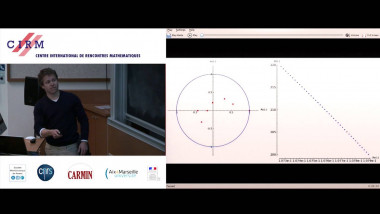
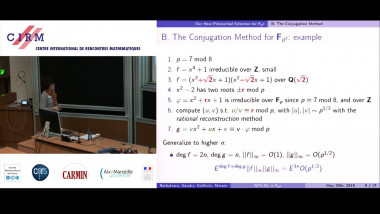
Let $\alpha$ $\epsilon$ $\mathbb{R}^d$ be a vector whose entries $\alpha_1, . . . , \alpha_d$ and $1$ are linearly independent over the rationals. We say that $S \subset \mathbb{T}^d$ is a bounded remainder set for the sequence of irrational rotations $\lbrace n\alpha\rbrace_{n\geqslant1}$ if the discrepancy $ \sum_{k=1}^{N}1_S (\lbrace k\alpha\rbrace) - N$ $mes(S)$ is bounded in absolute value as $N \to \infty$. In one dimension, Hecke, Ostrowski and Kesten characterized the intervals with this property. We will discuss the bounded remainder property for sets in higher dimensions. In particular, we will see that parallelotopes spanned by vectors in $\mathbb{Z}\alpha + \mathbb{Z}^d$ have bounded remainder. Moreover, we show that this condition can be established by exploiting a connection between irrational rotation on $\mathbb{T}^d$ and certain cut-and-project sets. If time allows, we will discuss bounded remainder sets for the continuous irrational rotation $\lbrace t \alpha : t$ $\epsilon$ $\mathbb{R}^+\rbrace$ in two dimensions.










































































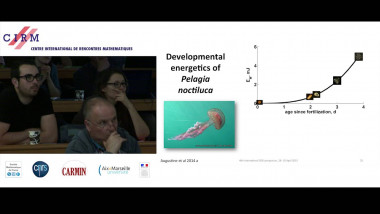
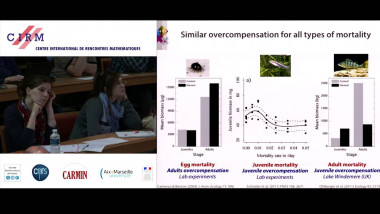
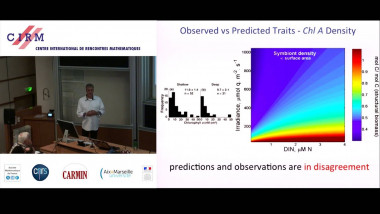





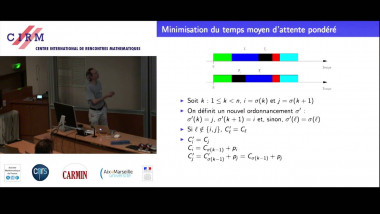


















![$k$-sum free sets in $[0,1]$](/media/cache/video_light/uploads/video/2015-09-10_de_Roton-video--19cf70874d3c5af7378c268cab865b06.jpg)