Rigidity theorems for the diffeomorphism action on spaces of metrics of positive scalar curvature
The diffeomorphism group $\mathrm{Diff}(M)$ of a closed manifold acts on the space $\mathcal{R}^+ (M)$ of positive scalar curvature metrics. For a basepoint $g$, we obtain an orbit map $\sigma_g: \mathrm{Diff}(M) \to \mathcal{R}^+(M)$ which induces a map $$(\sigma_g)_*:\pi_*( \mathrm{Diff}(M))\to\pi_{\ast}(\mathcal{R}^+(M))$$ on homotopy groups.
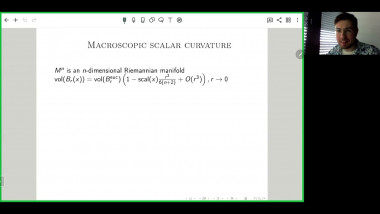
The rigidity theorems from the title assert that suitable versions of the map $(\sigma_g)_*$ factor through certain bordism groups. A special case of our main result asserts that $(\sigma_g)_*$ has finite image if $M$ is simply connected, stably parallelizable and of dimension at least $6$.
The results of this talk are from joint work of the speaker with Oscar Randal-Williams.