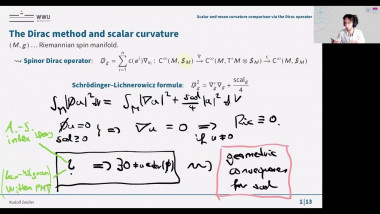
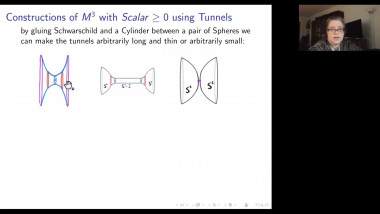
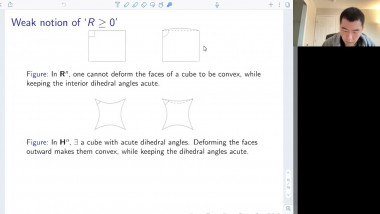
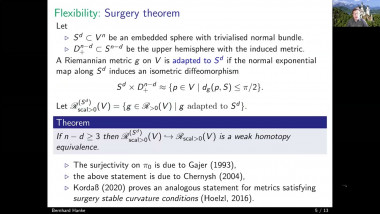
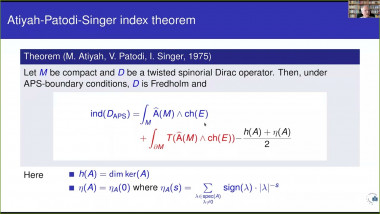
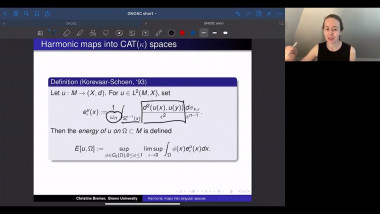
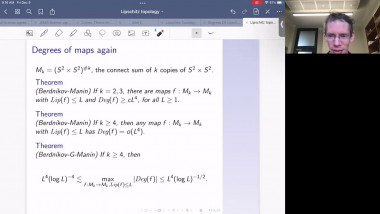
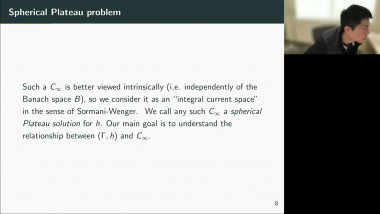
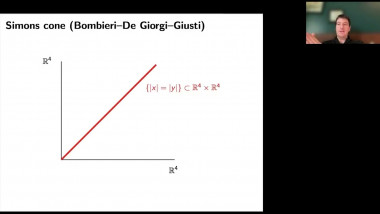
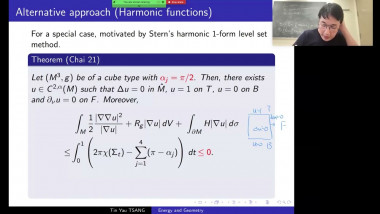
Spherical Plateau problem and applications
By Antoine Song
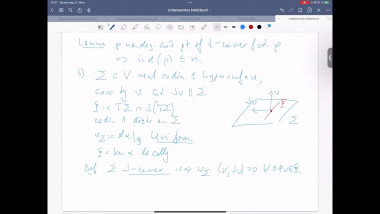
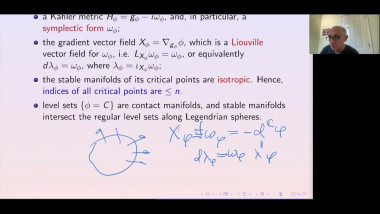
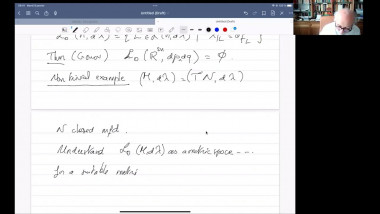
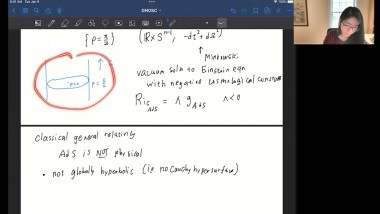
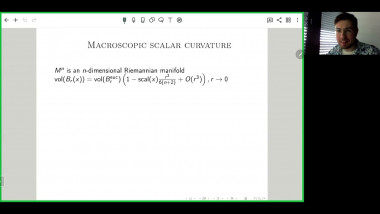
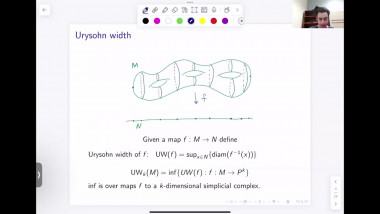
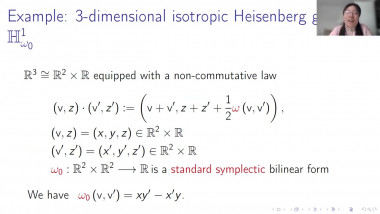
I will discuss an area minimization problem in certain quotients of the Hilbert sphere by countable groups. An early version of that setting appears in Besson-Courtois-Gallot’s work on the entropy inequality. As an application of this minimization problem, we obtain some stability results. For instance, consider a closed surface of genus at least $2$ endowed with a Riemannian metric $g$, and let $(S,g)$ be its universal cover. After normalizing $g$ so that the volume entropy of $(S,g)$ is $1$, it is well-known that the first eigenvalue $\lambda$ is at most $\frac14$, and equality holds if $g$ is a hyperbolic metric. The hyperbolic plane is in fact stable: if $\lambda$ is close to the upper bound $\frac14$, then $(S,g)$ is close to the hyperbolic plane in a Benjamini-Schramm topology.