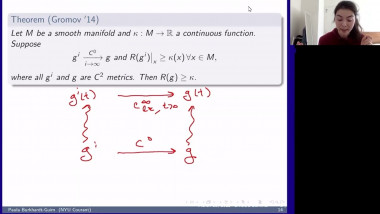
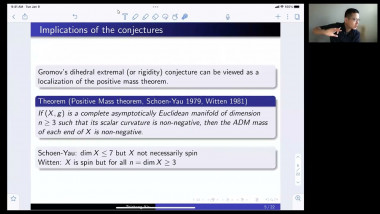
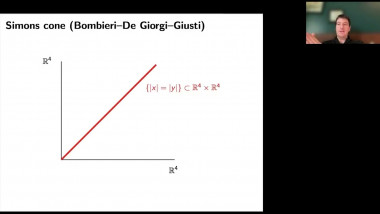
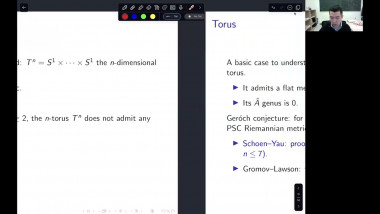
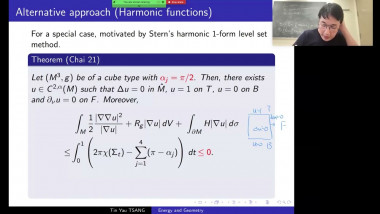
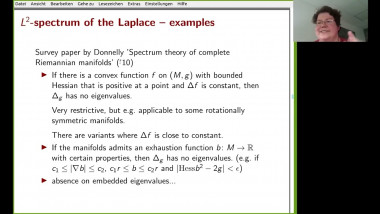
Recent inequalities on the mass-to-capacity ratio
By Pengzi Miao
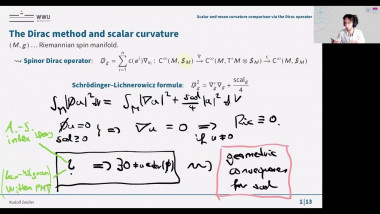
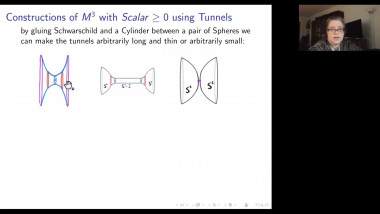
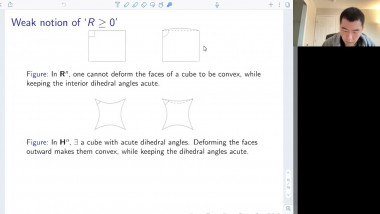
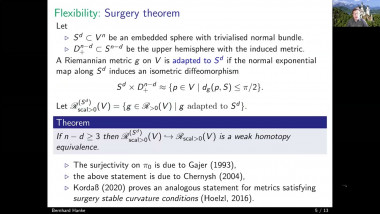
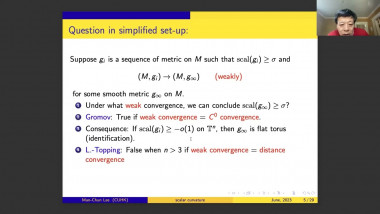
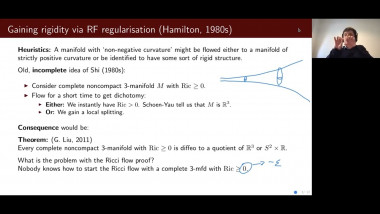
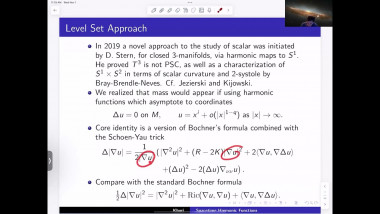
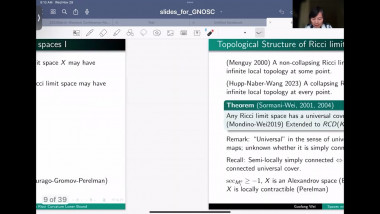
On an asymptotically flat 3-manifold, both the mass and the capacity have unit of length, and hence their ratio is a dimensionless quantity. In this talk, I will discuss recent work on establishing new inequalities for the mass-to-capacity ratio on manifolds with nonnegative scalar curvature. Besides revealing additional proofs of the positive mass theorem, applications of these inequalities include new sufficient conditions guaranteeing positive mass via $C^0$-geometry of regions separating the boundary and the infinity. If time permits, a proposal to study manifolds with the mass-to-capacity ratio bounded by one will also be discussed.