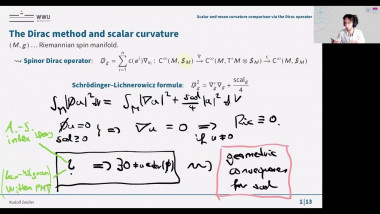
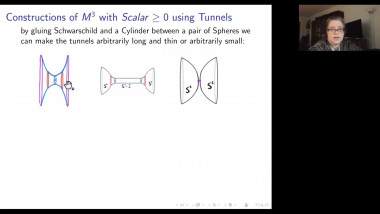
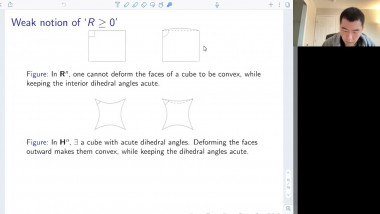
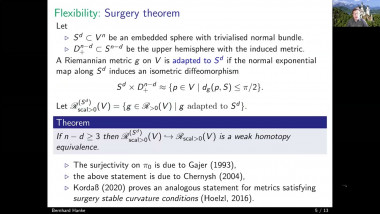
Self-similar solutions to extension and approximation problems
By Robert Young
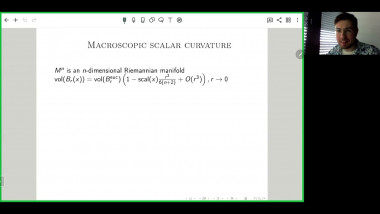
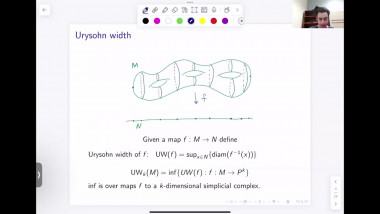
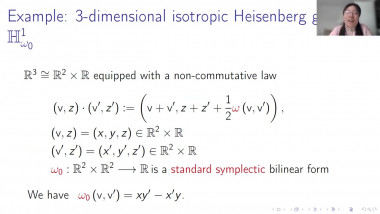
In 1979, Kaufman constructed a remarkable surjective Lipschitz map from a cube to a square whose derivative has rank 1 almost everywhere. In this talk, we will present some higher-dimensional generalizations of Kaufman's construction that lead to Lipschitz and Hölder maps with wild properties, including: topologically nontrivial maps from $S^m$ to $S^n$ with derivative of rank $n-1$, $\frac{2}{3}-\epsilon$--Hölder approximations of surfaces in the Heisenberg group, and Hölder maps from the disc to the disc that preserve signed area but approximate an arbitrary continuous map.