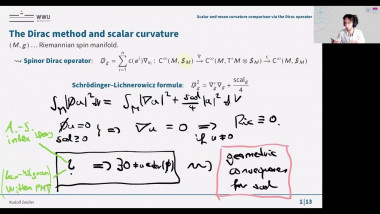
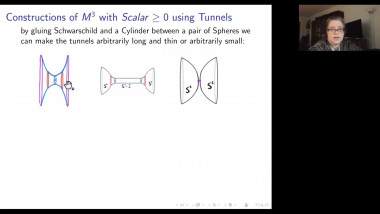
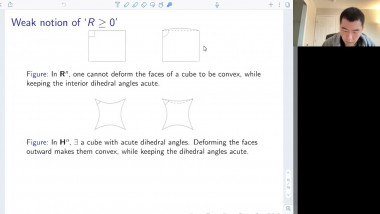
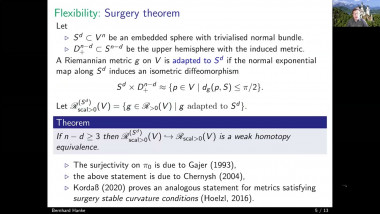
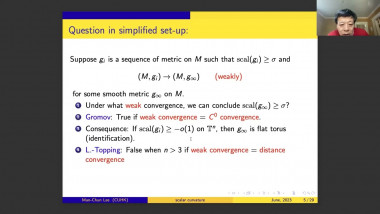
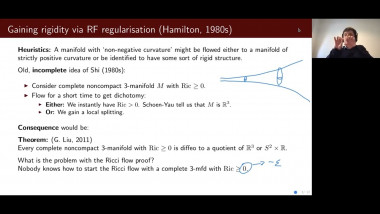
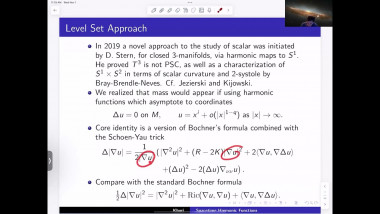
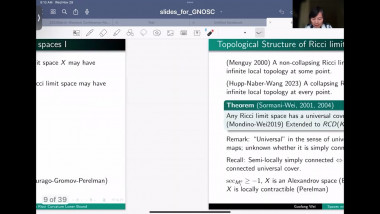
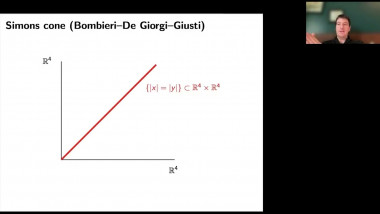
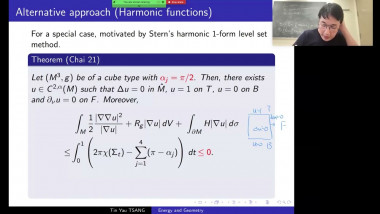
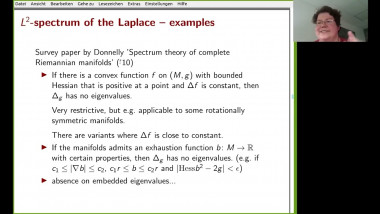
Logarithmic Sobolev inequalities on homogeneous spaces
By Langbing Luo
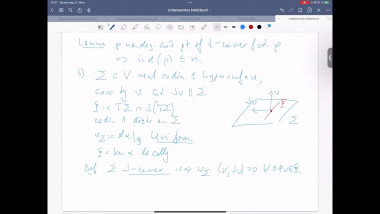
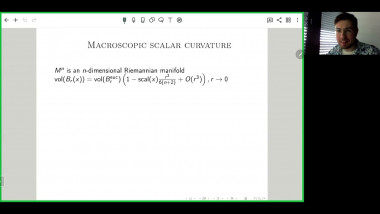
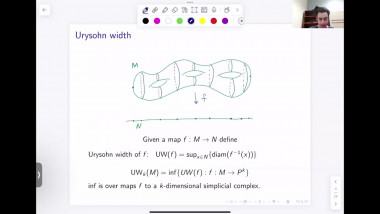
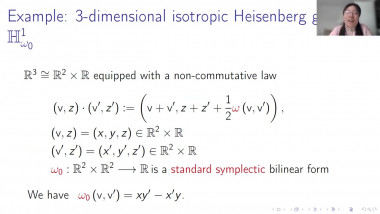
We consider sub-Riemannian manifolds which are homogeneous spaces equipped with a sub-Riemannian structure induced by a transitive action by a Lie group. The corresponding sub-Laplacian there is not an elliptic but a hypoelliptic operator. We study logarithmic Sobolev inequalities and show that the logarithmic Sobolev constant can be chosen to depend only on the Lie group acting transitively on such a space but the constant is independent of the action of its isotropy group. This approach allows us to track the dependence of the logarithmic Sobolev constant on the geometry of the underlying space, in particular we show that the constant is independent of the dimension of the underlying spaces in several examples. Based on joint work with M.Gordina.