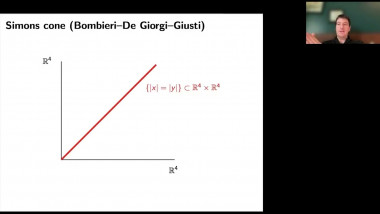
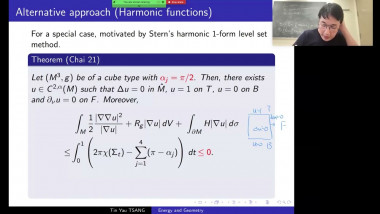
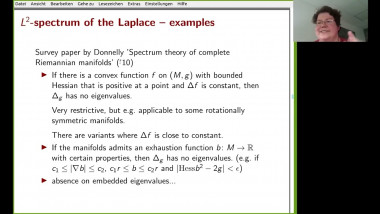
Macroscopic scalar curvature and volume
By Roman Sauer
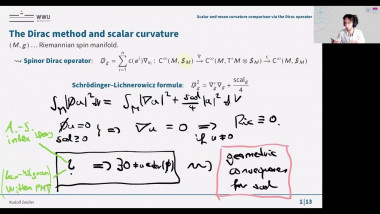
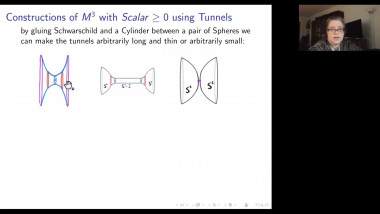
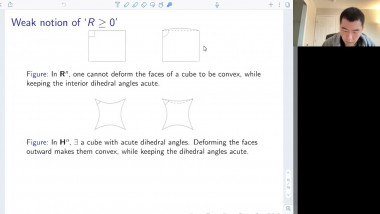
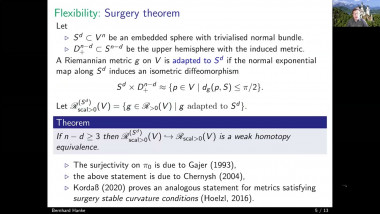
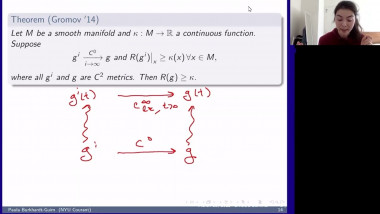
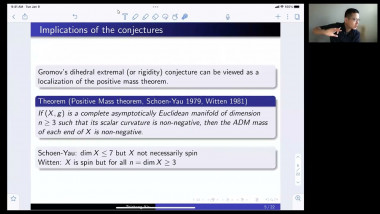
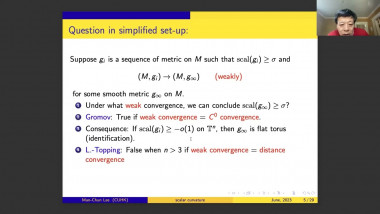
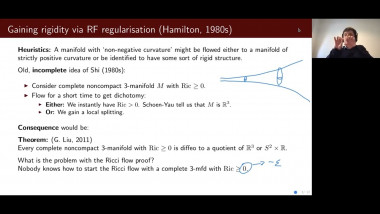
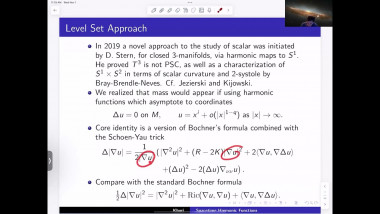
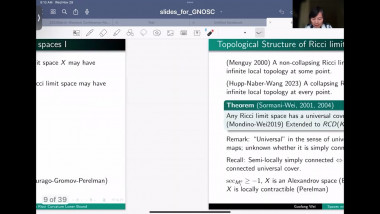
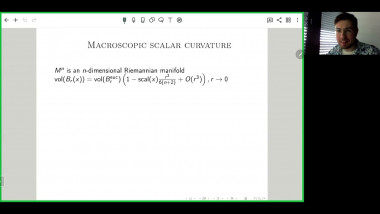
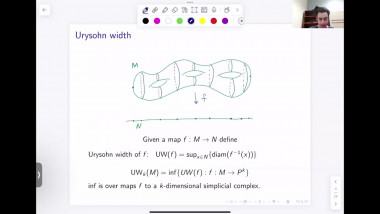
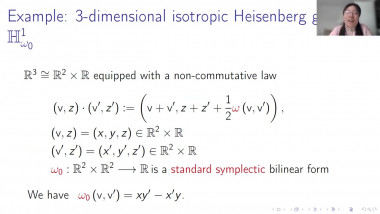
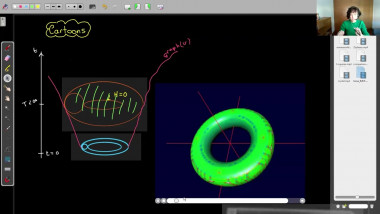
We prove a generalization of Gromov’s main inequality between volume and simplicial volume where the lower Ricci curvature bound is replaced by a macroscopic scalar curvature bound. This generalization, which is based on joint work with Sabine Braun, also extends a theorem of Guth from hyperbolic manifolds to arbitrary Riemannian manifolds. A quantitative version was more recently obtained by Hannah Alpert, which will also be presented in the talk.