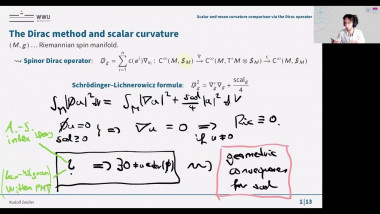
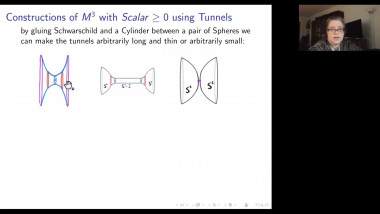
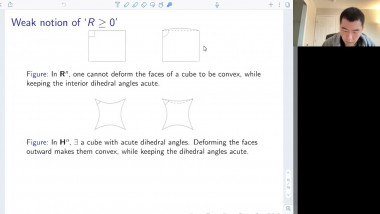
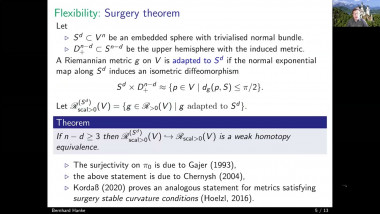
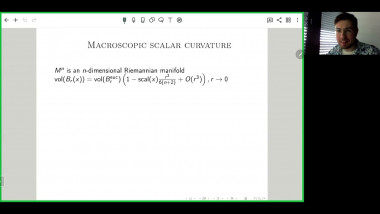
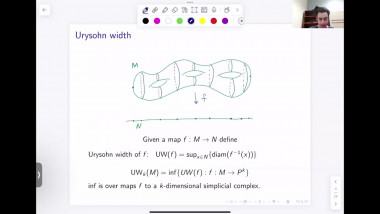
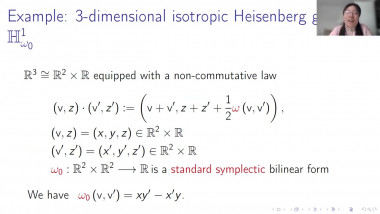
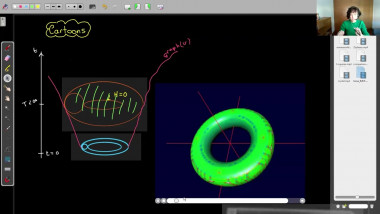
Nonnegative Ricci curvature, nilpotency, and Hausdorff dimension
By Jiayin Pan
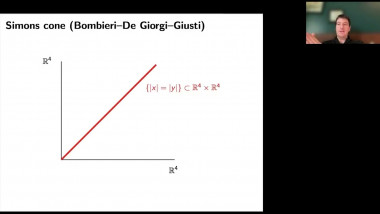
Continuing Wei's presentation, we will talk about how the universal covers of Nabonnand-type examples came to our attention. One geometric feature in these examples is that the minimal representing loops of $\pi_1(M,p)$ must escape from any bounded sets. This leads to wild limit orbits in the asymptotic cones of $\widetilde{M}$: these orbits are not convex and have large Hausdorff dimension. Then we will discuss the relations in general among the above-mentioned escape phenomenon, orbits in the asymptotic cones, and the virtual abelianness / nilpotency of the fundamental groups.