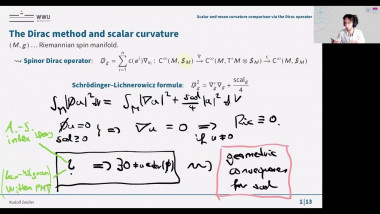
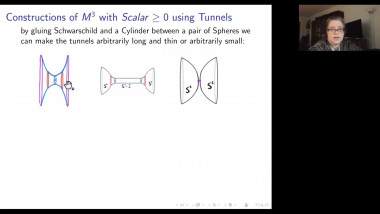
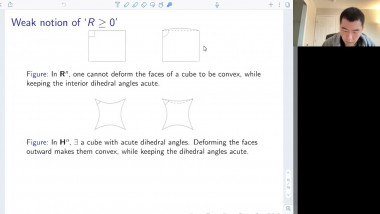
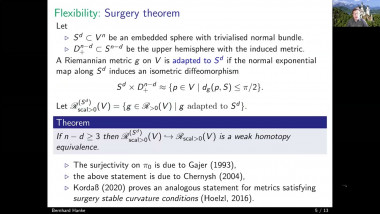
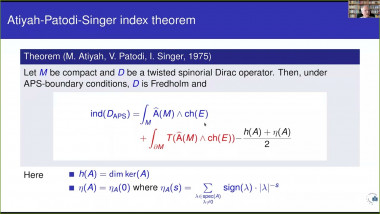
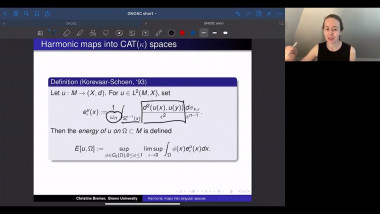
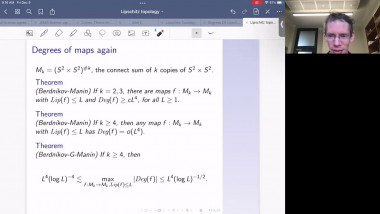
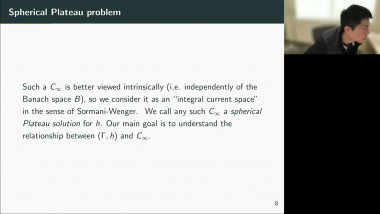
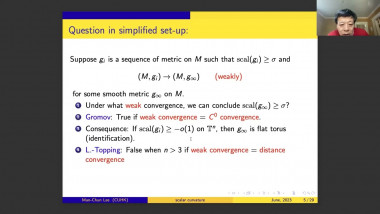
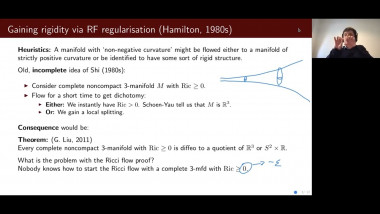
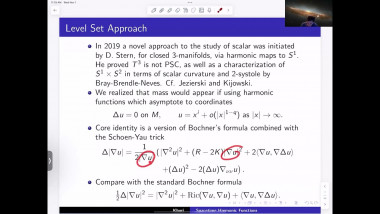
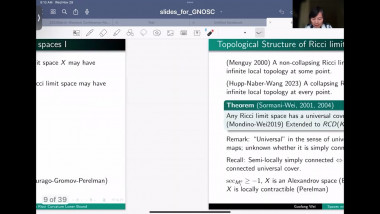
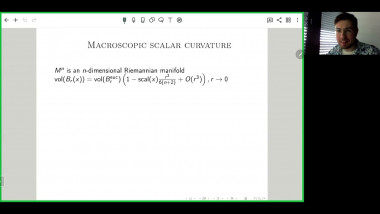
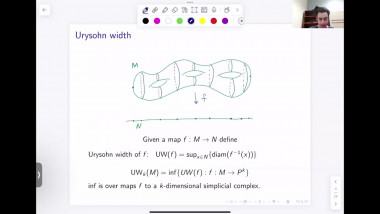
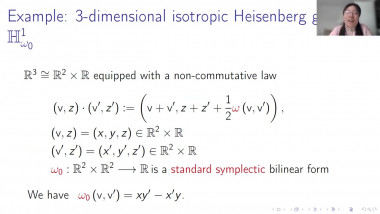
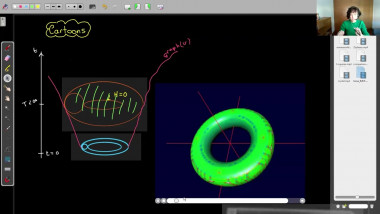
Scalar curvature rigidity and extremality in dimension 4
In this talk, I will discuss the Finsler--Thorpe trick for curvature operators in dimension 4, and how it can be combined with twisted spinor methods to show that large classes of compact 4-manifolds, with or without boundary, with nonnegative sectional curvature are area-extremal for scalar curvature. These techniques also show that any region of positive sectional curvature on a 4-manifold is locally area-extremal. This is joint work with McFeely Jackson Goodman (UC Berkeley).