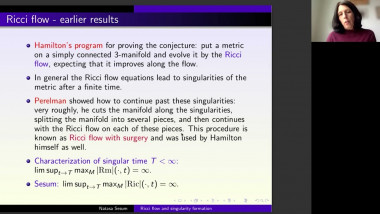
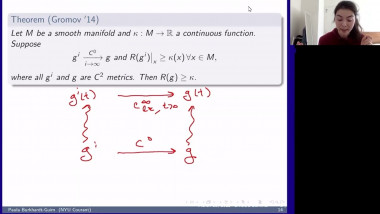
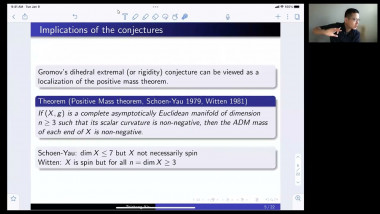
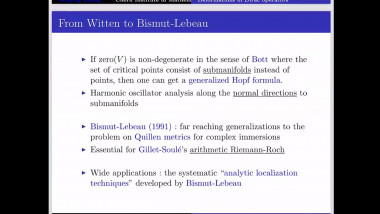
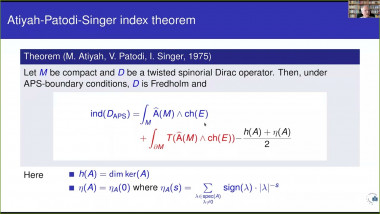
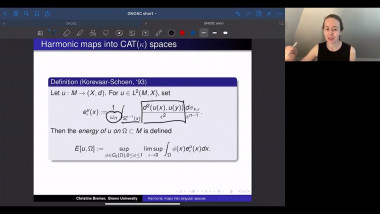
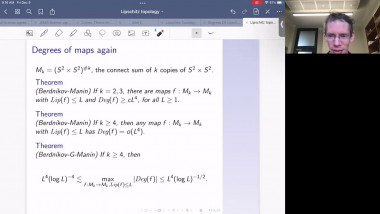
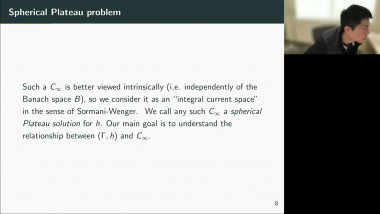
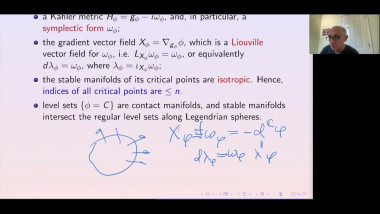
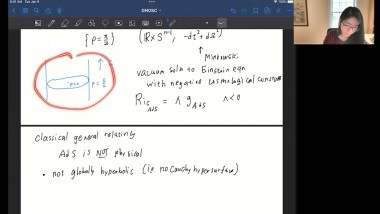
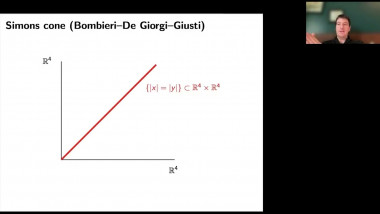
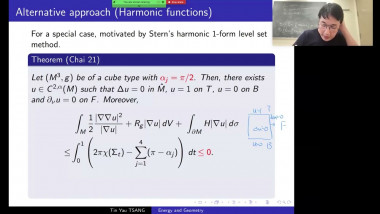
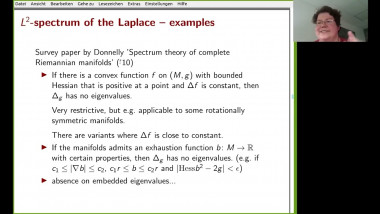
Waists measured via Urysohn width
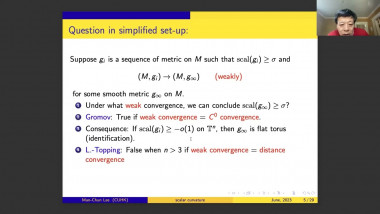
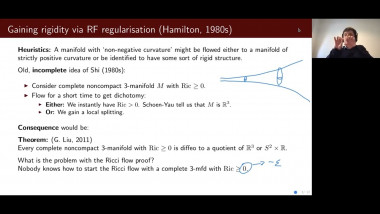
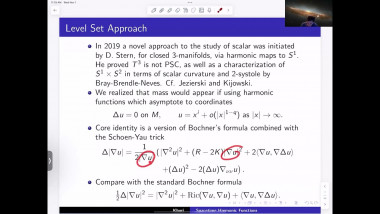
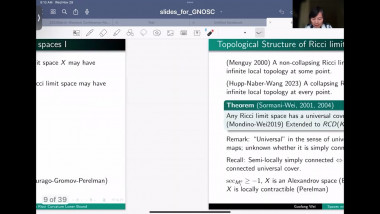
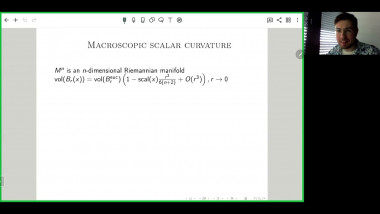
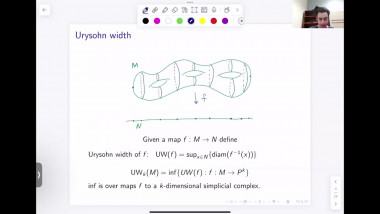
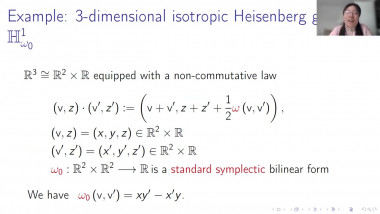
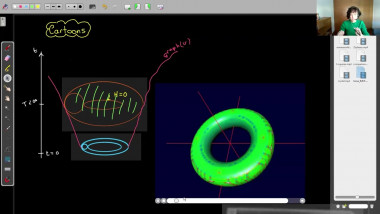
The Urysohn width measures the "approximate dimension" of a riemannian manifold by approximating it with a lower-dimensional simplicial complex. Positive scalar curvature conjecturally implies upper bounds on the width. An inductive approach to this conjecture and related ones requires understanding of the following question: If our manifold is sliced into chunks of small approximate dimension, does that imply that the manifold itself has controlled approximate dimension? I will explain a few results in that direction, mostly of negative nature.