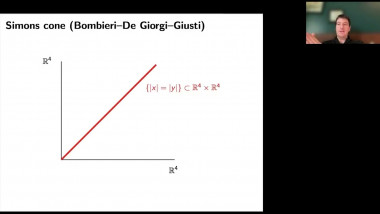
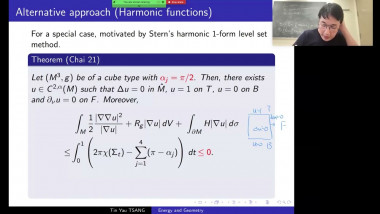
Intrinsic flat stability of Llarull’s theorem in dimension three
By Brian Allen
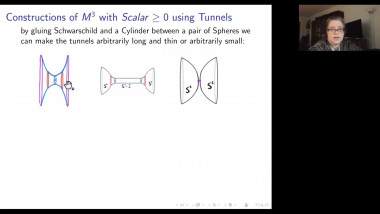
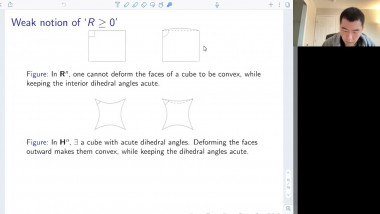
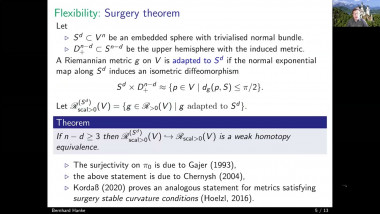
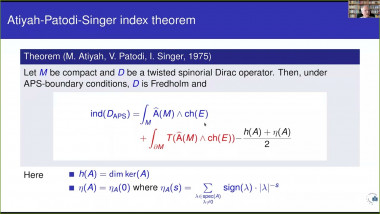
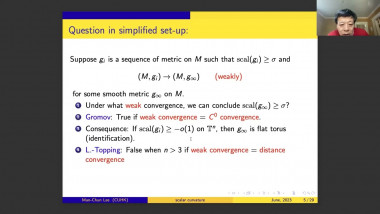
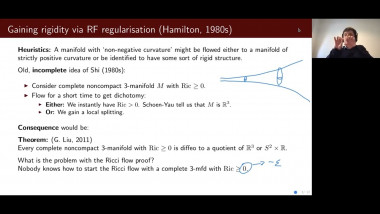
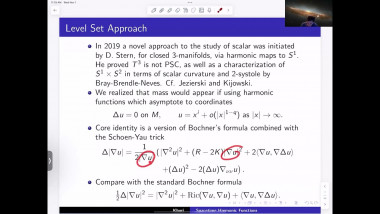
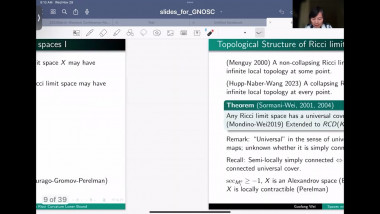
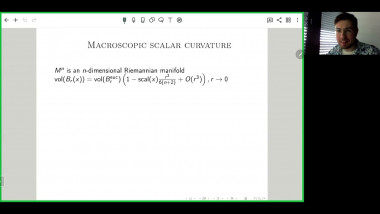
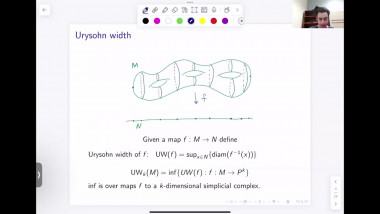
Llarull's Theorem states that any Riemannian metric on the $n$-sphere which has scalar curvature greater than or equal to $n(n-1)$, and whose distance function is bounded below by the unit sphere's, is isometric to the unit sphere. Gromov later posed the Spherical Stability Problem, probing the flexibility of this fact, which we give a resolution of in dimension $3$. We show that a sequence of Riemannian $3$-spheres almost satisfying the hypotheses of Llarull's theorem with uniformly bounded Cheeger isoperimetric constant must approach the round $3$-sphere in the volume preserving Sormani-Wenger Intrinsic Flat sense. The argument is based on a proof of Llarull's Theorem due to Hirsch-Kazaras-Khuri-Zhang (https://arxiv.org/abs/2209.12857) using spacetime harmonic functions and a characterization of Sormani-Wenger Intrinsic Flat convergence given by Allen-Perales-Sormani (https://arxiv.org/abs/2003.01172). This is joint work with E. Bryden and D. Kazaras (https://arxiv.org/abs/2305.18567).