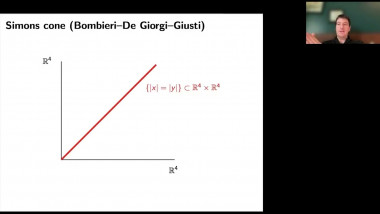
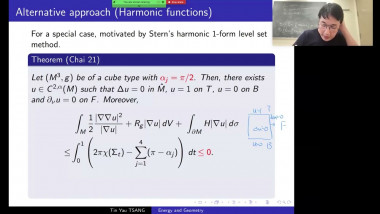
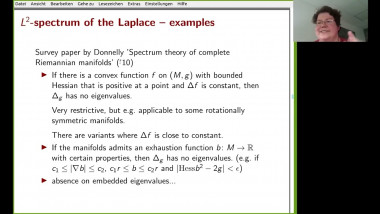
Ricci curvature, fundamental group and the Milnor conjecture (I)
By Aaron Naber
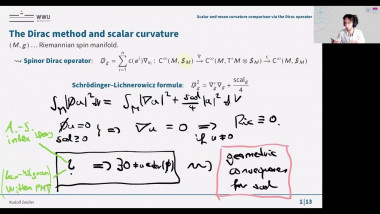
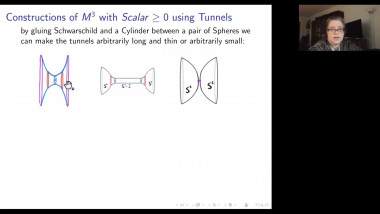
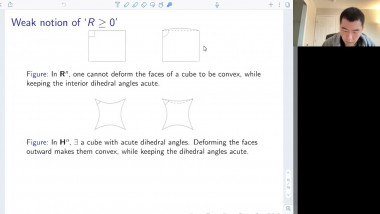
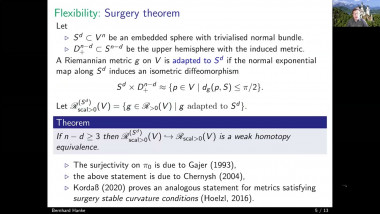
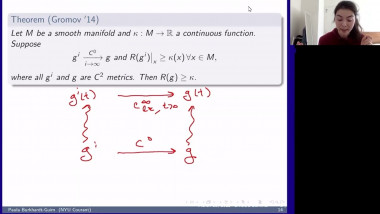
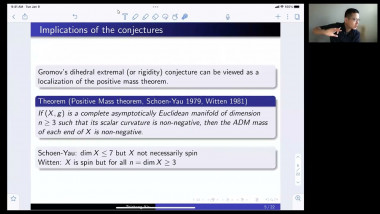
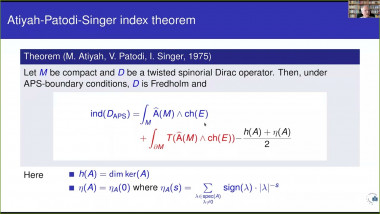
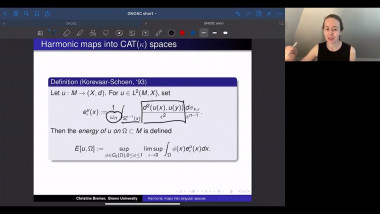
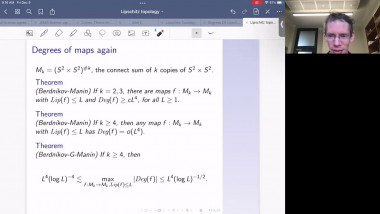
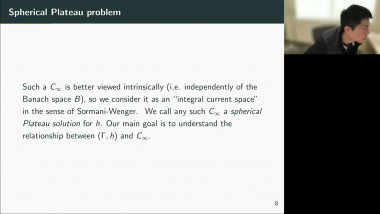
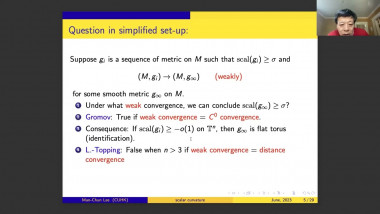
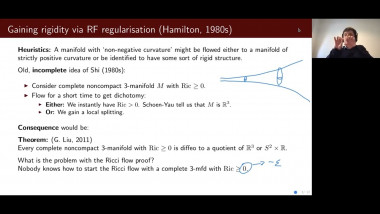
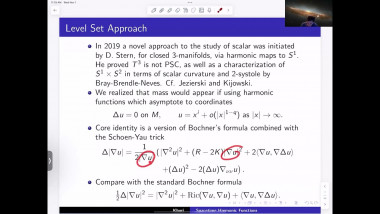
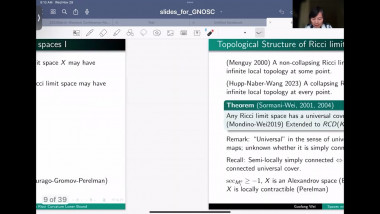
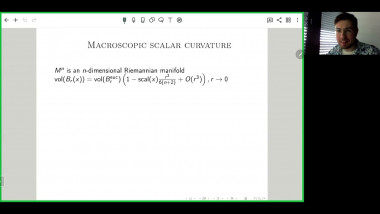
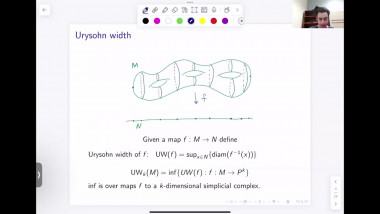
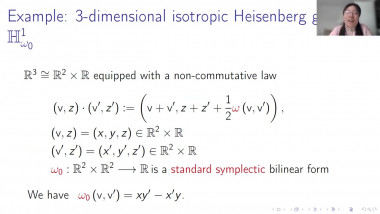
It was conjectured by Milnor in 1968 that the fundamental group of a complete manifold with nonnegative Ricci curvature is finitely generated. In this talk we will discuss a counterexample, which provides an example $M^7$ with $\mathrm{Ric}>= 0$ such that $\pi_1(M)=Q/Z$ is infinitely generated. There are several new points behind the result. The first is a new topological construction for building manifolds with infinitely generated fundamental groups, which can be interpreted as a smooth version of the fractal snowflake. The ability to build such a fractal structure will rely on a very twisted gluing mechanism. Thus the other new point is a careful analysis of the mapping class group $\pi_0(\mathrm{Diff}(S^3\times S^3))$ and its relationship to Ricci curvature. In particular, a key point will be to show that the action of $\pi_0(\mathrm{Diff}(S^3\times S^3))$ on the standard metric $g_{S^3\times S^3}$ lives in a path connected component of the space of metrics with $\mathrm{Ric}>0$.