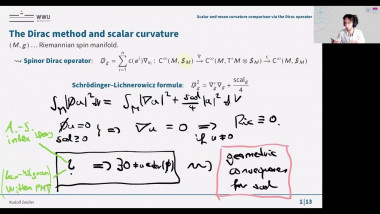
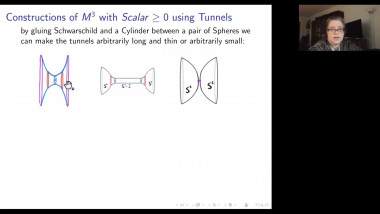
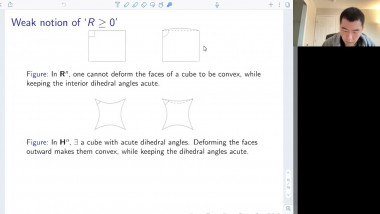
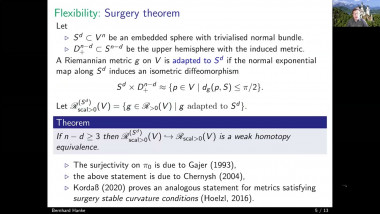
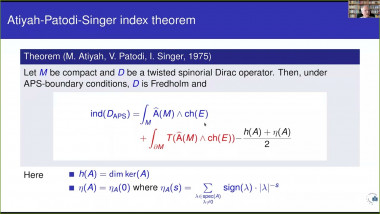
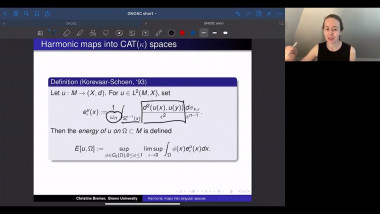
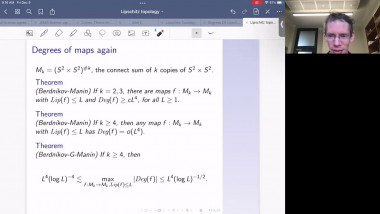
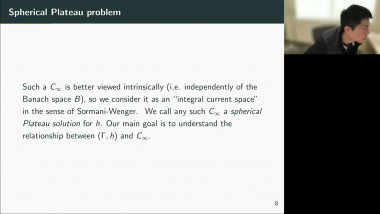
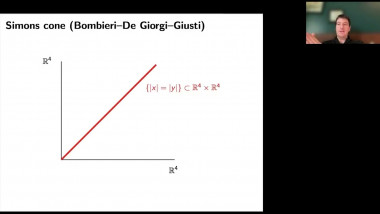
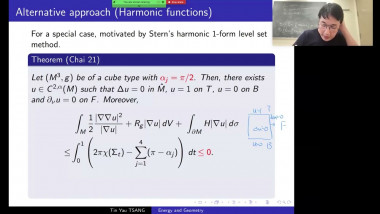
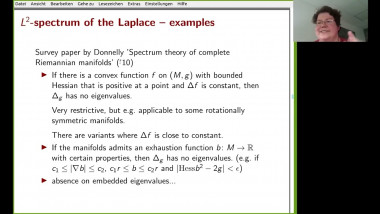
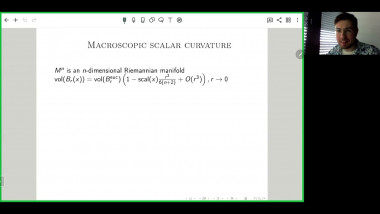
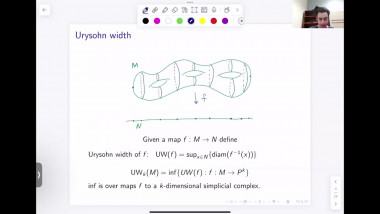
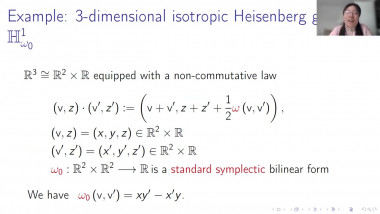
Rigidity results for initial data sets satisfying the dominant energy condition
In this talk, I will discuss rigidity theorems for initial data sets (IDS) on compact, smooth spin manifolds with boundary and on compact convex polytopes, both under the dominant energy condition (DEC). For manifolds with smooth boundary, by studying a boundary value problem for Dirac operators, we identify a natural class of smooth manifolds with boundary as extremal cases of IDS satisfying the DEC. For convex polytopes, we extend Gromov’s polytope comparison framework for positive scalar curvature, adapting it to IDS satisfying the DEC through approximations by manifolds with smooth boundary. These results are based on joint work with C. Bär, S. Brendle, and B. Hanke.