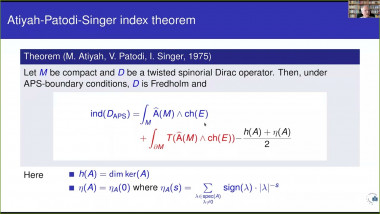
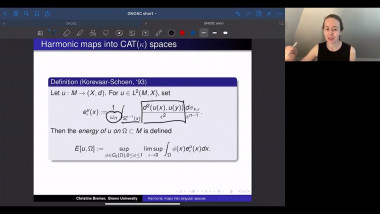
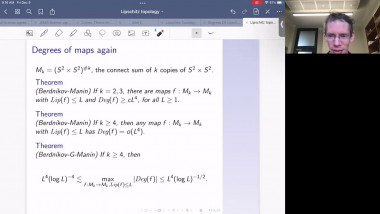
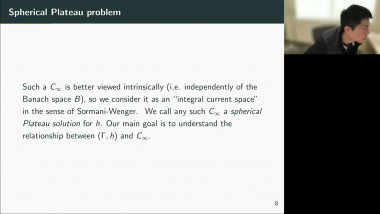
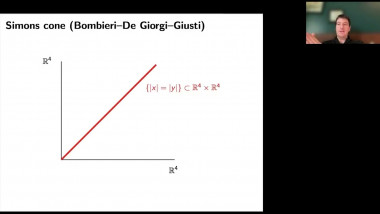
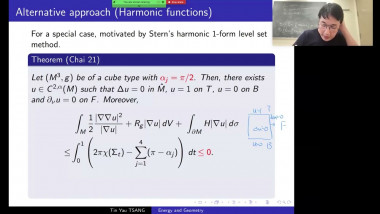
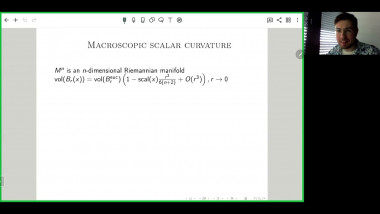
Recent developments in constant mean curvature hypersurfaces II
Continuing from the previous talk, we will first discuss two min-max theorems for constructing prescribed mean curvature hypersurfaces in non-compact spaces. The first concerns the existence of prescribed mean curvature hypersurfaces in Euclidean space, and the second concerns the existence of constant mean curvature hypersurfaces in asymptotically flat manifolds. Following this, we will introduce the half-volume spectrum of a manifold $M$. This is analogous to the usual volume spectrum, except that we restrict to p-sweepouts whose slices are each required to enclose half the volume of $M$. We use the Allen-Cahn min-max theory to find hypersurfaces associated to the half-volume spectrum. Each hypersurface consists of a constant mean curvature component enclosing half the volume of $M$ plus a (possibly empty) collection of minimal components.