Carmin.tv

Mathématiques
et Interactions

Merci de nous donner votre avis pour que nous puissions améliorer votre expérience !
Albert Schwarz : Quantum Mechanics and Quantum Field Theory from Algebraic and Geometric Viewpoints
3 videos

Albert Schwarz : Quantum Mechanics and Quantum Field Theory from Algebraic and Geometric Viewpoints

Not Only Scalar Curvature Seminar
60 videos

Not Only Scalar Curvature Seminar

Clément Delcamp : Topological Symmetry and Duality in Quantum Lattice Models
4 videos

Clément Delcamp : Topological Symmetry and Duality in Quantum Lattice Models

Aggregation-Diffusion Equations & Collective Behavior: Analysis, Numerics and Applications / Conférence Chaire Jean Morlet: Equations d'agrégation-diffusion et comportement collectif: Analyse, schémas numériques et applications
5 videos

Aggregation-Diffusion Equations & Collective Behavior: Analysis, Numerics and Applications / Conférence Chaire Jean Morlet: Equations d'agrégation-diffusion et comportement collectif: Analyse, schémas numériques et applications

Toutes les collections
CIMPA
CIRM
IHES
IHP
LMR
SMF
Tous les instituts
Combinatorics and Arithmetic for Physics: special days 2023

Collection Combinatorics and Arithmetic for Physics: special days 2023

Organisateur(s) Gérard H. E. DUCHAMP, Maxim KONTSEVICH, Gleb KOSHEVOY, Sergei NECHAEV and Karol A. PENSON
Date(s) 15/11/2023 - 17/11/2023
URL associée https://www-lipn.univ-paris13.fr/~duchamp/Conferences/CAP10_2023.html
00:00:00 / 00:00:00
9 27

The miracle of integer eigenvalues

De Maxim Kontsevich

Apparaît également dans la collection : Maxim Kontsevich

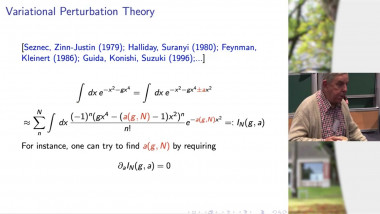
One can associate with any finite poset with N elements a square matrix depending on 2(N − 1) variables, such that each matrix coefficient is just one of variables. Remarkably, all eigenvalues of this matrix are integer linear combinations of variables. Even in the case of the trivial poset, the structure of eigenvalues gives a new result concerning representations of the symmetric group. This is a joint work with R.Kenyon, O.Ogievetsky, A.Pohoata, W.Sawin and S.Shlosman.

Informations sur la vidéo

  • Date de captation 15/11/2023
  • Date de publication 20/11/2023
  • Institut IHES
  • Langue Anglais
  • Audience Chercheurs
  • Format MP4

Domaine(s)

Dernières questions liées sur MathOverflow

Pour poser une question, votre compte Carmin.tv doit être connecté à mathoverflow

Poser une question sur MathOverflow




Inscrivez-vous

  • Mettez des vidéos en favori
  • Ajoutez des vidéos à regarder plus tard &
    conservez votre historique de consultation
  • Commentez avec la communauté
    scientifique
  • Recevez des notifications de mise à jour
    de vos sujets favoris
Copyright Carmin.tv 2024
En poursuivant votre navigation sur ce site, vous acceptez l'utilisation de cookies pour améliorer votre expérience. En savoir plus sur notre politique de confidentialité.
Accepter
Donner son avis