Carmin.tv

Mathématiques
et Interactions

Merci de nous donner votre avis pour que nous puissions améliorer votre expérience !
Not Only Scalar Curvature Seminar
60 videos

Not Only Scalar Curvature Seminar

Albert Schwarz : Quantum Mechanics and Quantum Field Theory from Algebraic and Geometric Viewpoints
2 videos

Albert Schwarz : Quantum Mechanics and Quantum Field Theory from Algebraic and Geometric Viewpoints

Clément Delcamp : Topological Symmetry and Duality in Quantum Lattice Models
4 videos

Clément Delcamp : Topological Symmetry and Duality in Quantum Lattice Models

Aggregation-Diffusion Equations & Collective Behavior: Analysis, Numerics and Applications / Conférence Chaire Jean Morlet: Equations d'agrégation-diffusion et comportement collectif: Analyse, schémas numériques et applications
5 videos

Aggregation-Diffusion Equations & Collective Behavior: Analysis, Numerics and Applications / Conférence Chaire Jean Morlet: Equations d'agrégation-diffusion et comportement collectif: Analyse, schémas numériques et applications

Toutes les collections
CIMPA
CIRM
IHES
IHP
LMR
SMF
Tous les instituts
Combinatorics and Arithmetic for Physics: special days 2023

Collection Combinatorics and Arithmetic for Physics: special days 2023

Organisateur(s) Gérard H. E. DUCHAMP, Maxim KONTSEVICH, Gleb KOSHEVOY, Sergei NECHAEV and Karol A. PENSON
Date(s) 15/11/2023 - 17/11/2023
URL associée https://www-lipn.univ-paris13.fr/~duchamp/Conferences/CAP10_2023.html
00:00:00 / 00:00:00
14 27

New Initial Approximation in Loop Vertex Expansion

De Vasily Sazonov

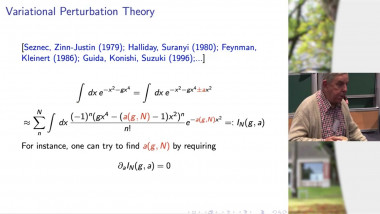
Loop Vertex Expansion (LVE) was developed for the construction of QFT models with local and non-local interactions. Using LVE, one can prove the analyticity in the finite cardioid-like domain in the complex plain of the coupling constant of the free energies and cumulants of various vector, matrix, or tensor-type models. In this talk, applying the idea of choosing the initial approximation depending on the coupling constant, I construct the analytic continuation of the free energy of the quartic matrix model beyond the standard LVE cardioid.

Informations sur la vidéo

  • Date de captation 16/11/2023
  • Date de publication 22/11/2023
  • Institut IHES
  • Langue Anglais
  • Audience Chercheurs
  • Format MP4

Domaine(s)

Dernières questions liées sur MathOverflow

Pour poser une question, votre compte Carmin.tv doit être connecté à mathoverflow

Poser une question sur MathOverflow




Inscrivez-vous

  • Mettez des vidéos en favori
  • Ajoutez des vidéos à regarder plus tard &
    conservez votre historique de consultation
  • Commentez avec la communauté
    scientifique
  • Recevez des notifications de mise à jour
    de vos sujets favoris
Copyright Carmin.tv 2024
En poursuivant votre navigation sur ce site, vous acceptez l'utilisation de cookies pour améliorer votre expérience. En savoir plus sur notre politique de confidentialité.
Accepter
Donner son avis