Calabi-Yau manifolds, mirror symmetry, and $F$-theory - part I
Also appears in collection : School on the Mathematics of String Theory / Ecole sur les mathématiques de la théorie des cordes
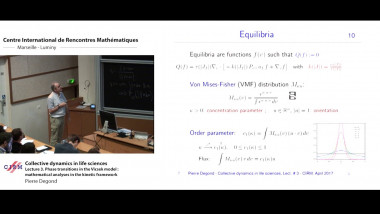
There are five superstring theories, all formulated in 9+1 spacetime dimensions; lower-dimensional theories are studied by taking some of the spatial dimensions to be compact (and small). One of the remarkable features of this setup is that the same lower-dimensional theory can often be realized by pairing different superstring theories with different geometries. The focus of these lectures will be on the mathematical implications of some of these physical “dualities.” Our main focus from the string theory side will be the superstring theories known as type IIA and type IIB. The duality phenomenon occurs for compact spaces of various dimensions and types. We will begin by discussing “T-duality” which uses tori as the compact spaces. We will then digress to introduce M-theory as a strong-coupling limit of the type IIA string theory, and F-theory as a variant of the type IIB string theory whose existence is motivated by T-duality. The next topic is compactifying the type IIA and IIB string theories on K3 surfaces (where the duality involves a change of geometric parameters but not a change of string theory). By the third lecture, we will have turned our attention to Calabi-Yau manifolds of higher dimension, and the “mirror symmetry” which relates pairs of them. Various aspects of mirror symmetry have various mathematical implications, and we will explain how these are conjecturally related to each other.