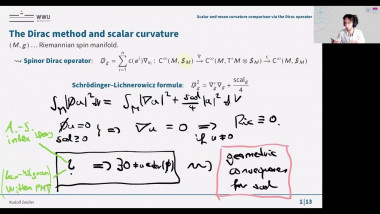
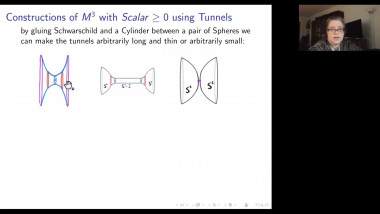
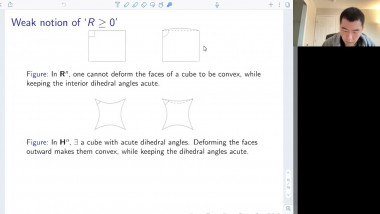
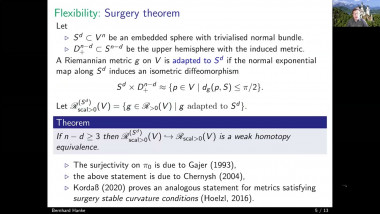
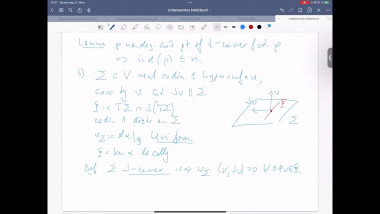
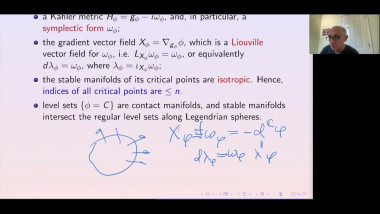
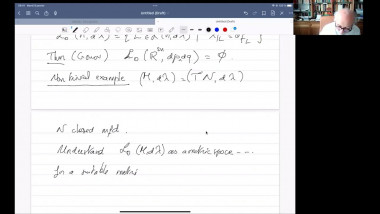
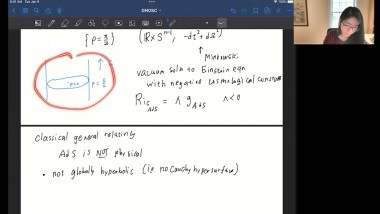
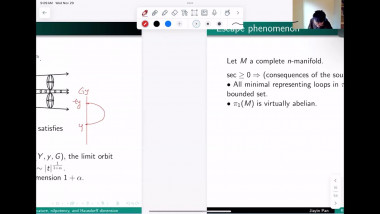
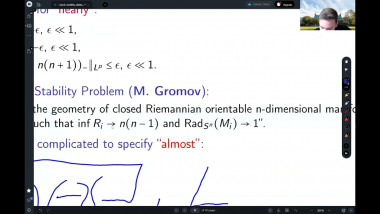
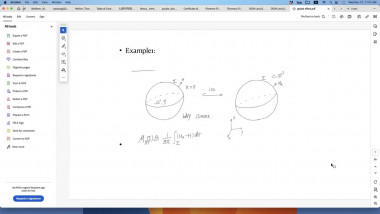
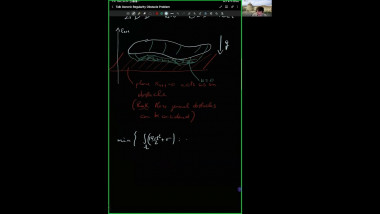
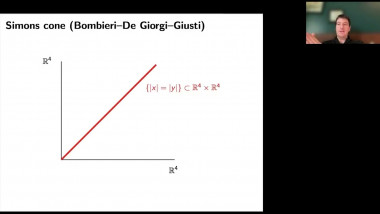
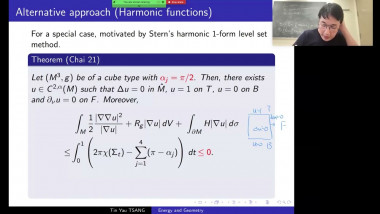
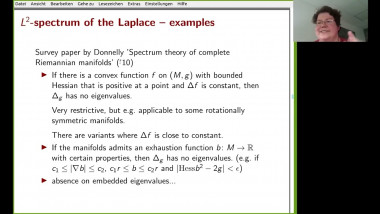
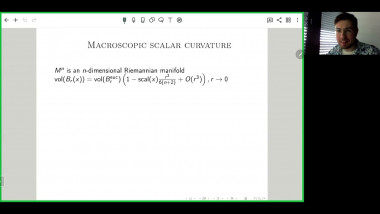
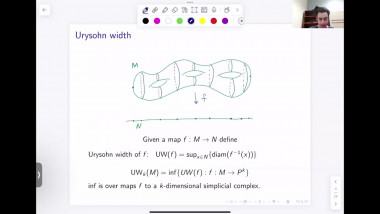
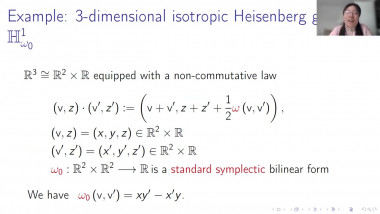
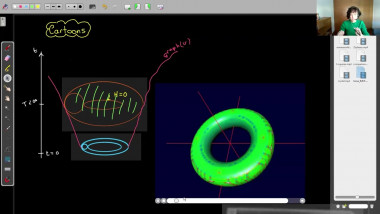
On the stability of spectra under coverings
Given a Riemannian covering $p\colon M\to N$ of closed Riemannian manifolds and a number $\Lambda>0$, are there eigenfunctions on $M$, with eigenvalue in $(0,\Lambda)$, which are not pull-backs of eigenfunctions on $N$? I will discuss this question and different answers (joint work with Sugata Mondal).