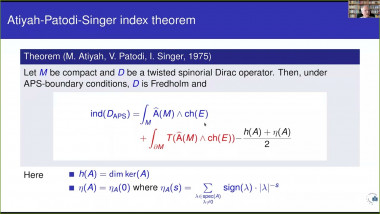
How hard is the theorem of Stolz?
De Fedor Manin
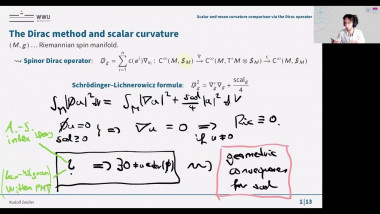
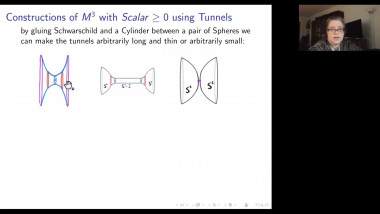
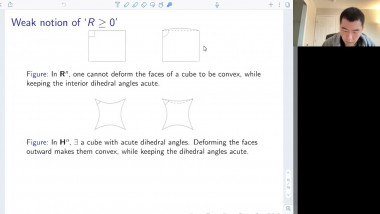
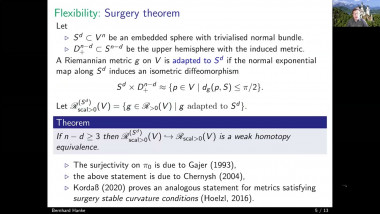
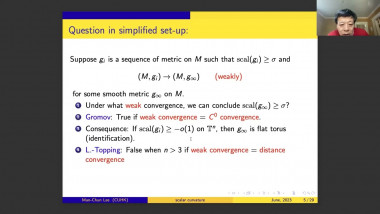
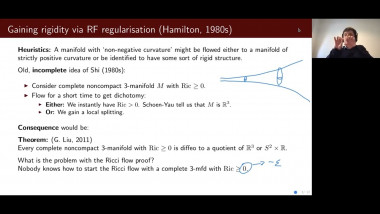
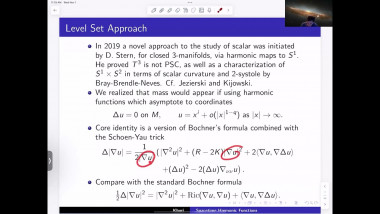
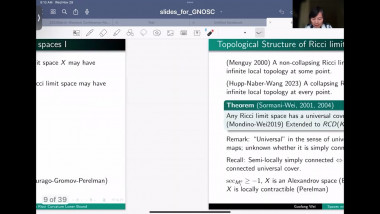
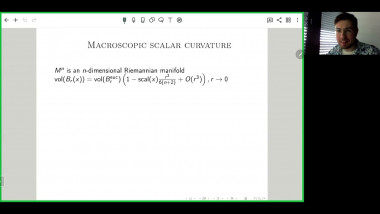
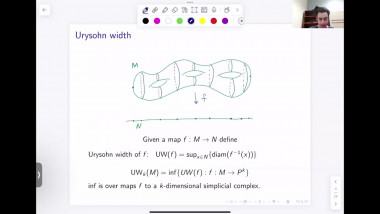
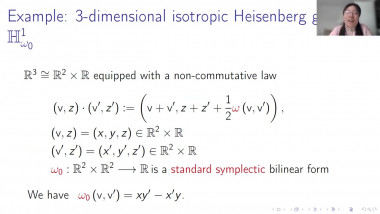
Stolz, building on work of Gromov–Lawson and Atiyah–Singer–Lichnerowicz–Hitchin, discovered exactly which simply connected spin manifolds have metrics of positive scalar curvature. Now say someone gives you a metric on a manifold which admits a metric of positive scalar curvature. How much more complicated do you have to make this metric so that it actually has positive scalar curvature? How hard is it to find a deformation between these two metrics? I will discuss joint work with Shmuel Weinberger, Zhizhang Xie and Guoliang Yu which gives some answers to these questions.