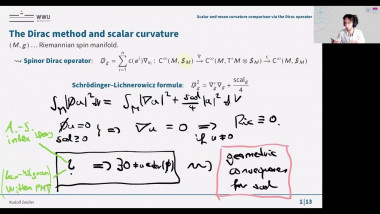
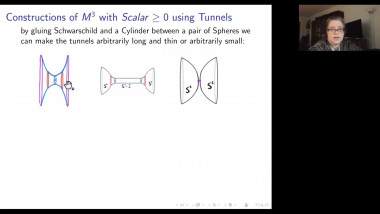
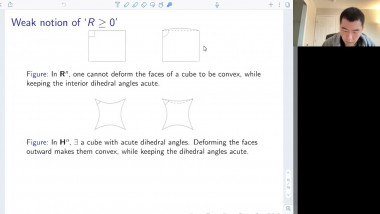
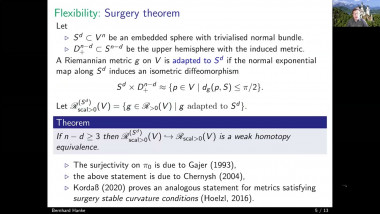
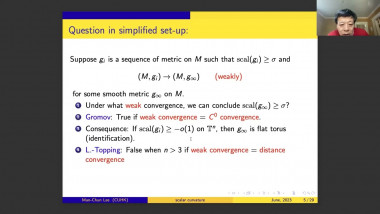
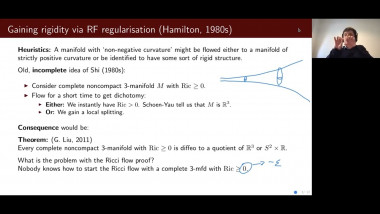
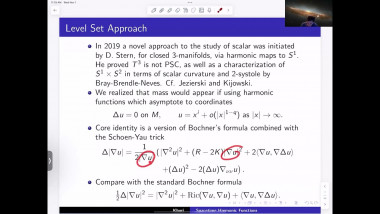
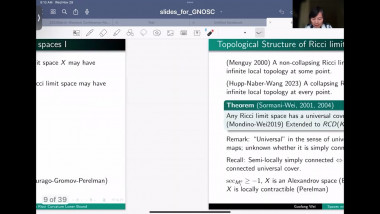
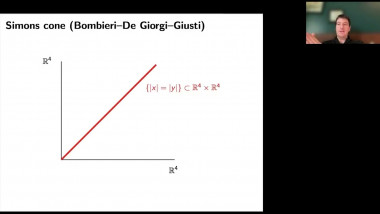
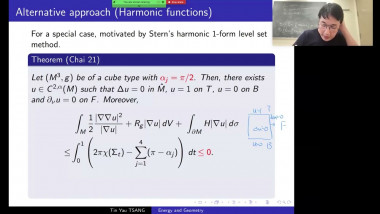
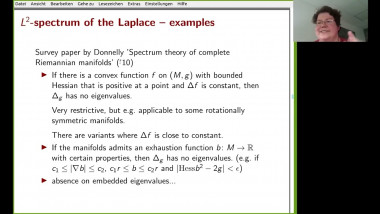
A higher dimensional generalization of the Birkhoff attractor
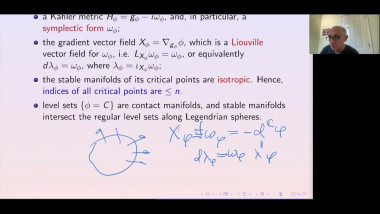
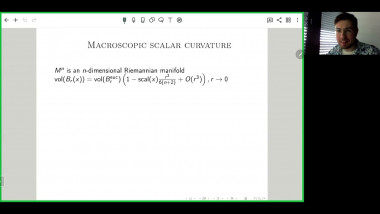
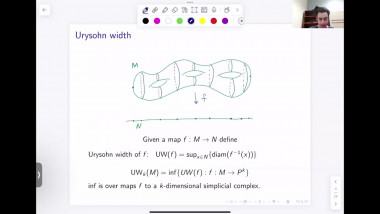
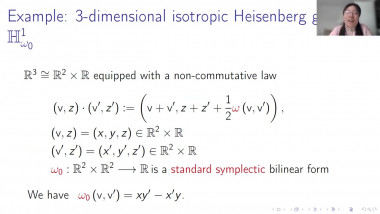
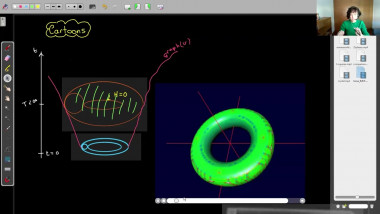
The spectral metric introduced in the talk of Claude Viterbo is a useful tool to study the dynamics of symplectic maps (in particular Hamiltonian systems). In this talk we will see that it can also be used to study the dynamics of conformal symplectic maps (in particular damped Hamiltonian systems). In particular, we will see how to generalize to higher dimension the classical Birkhoff attractor (1932) which was so far only defined in the $2$-dimensional annulus. This is based on joint work in progress with Marie-Claude Arnaud and Claude Viterbo.