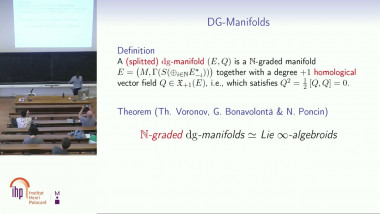
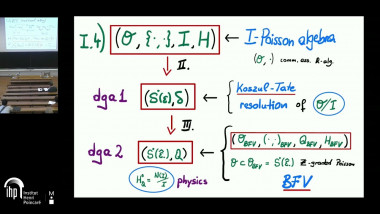
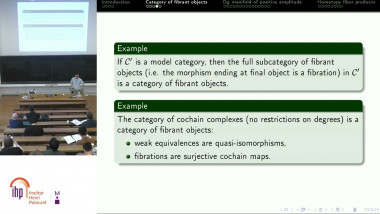
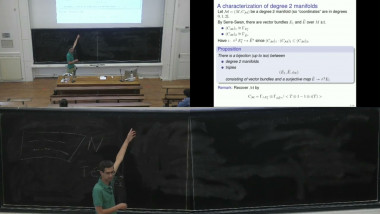
A main motivation for developing derived differential geometry is to deal with singularities arising from zero loci or intersections of submanifolds. Both zero loci and intersections can be considered as fiber products of manifolds which may not be manifolds. To deal with this issue, we extend the category of differentiable manifolds to the category of dg manifolds of finite positive amplitudes in which "homotopy fiber products" exist. In this talk, I would like to explain properties of this larger category (it is a category of fibrant objects) and our construction of homotopy fiber products of manifolds. The talk is mainly based on a joint work with Kai Behrend and Ping Xu.
De Pavel Mnev
De Jae-Suk Park
De Ruben Louis
De Thomas Strobl
De Hsuan-Yi Liao
De Christian Blohmann
De Tilmann Wurzbacher
De Owen Gwilliam
De Adrien Brochier
De Jérémie Pierard de Maujouy
De Pavol Severa
De Roberta Anna Iseppi
De Alain Connes
De Teresa Krick
De Teresa Krick
