Quantum exponentiation of Hamiltonian Poisson varieties
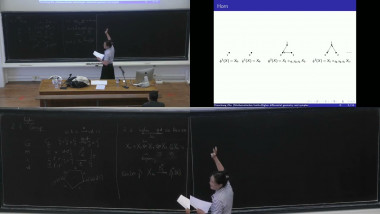
Let G be a complex reductive algebraic group. Various authors have developed notions of (quas G-Poisson varieties equipped with a multiplicative version of a moment map, valued in the group G. One of the main motivations for that formalism is that it provides a nice combinatorial description of the canonical Atiyah--Bott Poisson structure on character varieties of surfaces. A crucial feature of these structures is that they come equipped with certain operations (fusion and Hamiltonian reduction) which in particular expresses the compatibility of the Poisson structure on character varieties with cutting and gluing of surfaces.
Exponentiation is a procedure taking an ordinary Hamiltonian variety (i.e. a Poisson variety equipped with a moment map into the dual of the Lie algebra g of G) and turning it (formally) into a "multiplicative" one. Crucially, this operation is compatible with fusion and Hamiltonian reduction on both sides, showing that the character variety of a surface is formally Poisson isomorphic to a much simpler Poisson variety. All of these structures/operations have natural interpretations in the framework of shifted Poisson structures. Exponentation, in particular, comes from a certain formal isomorphism of 1-shifted Poisson stacks g^*/G -- G/G.
In this talk, I'll describe a quantization of this construction which is essentially given by pulling back along a certain monoidal functor from quantum to classical Harish-Chandra bimodules, which quantizes the above 1-shifted Poisson map. I'll then explain how this construction is compatible with categorical/quantum analogs of fusion and Hamiltonian reduction, and I'll present some applications.