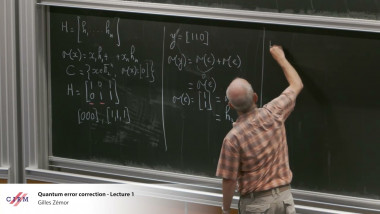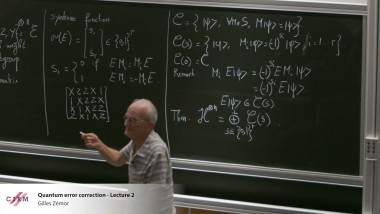On APN and AB power functions
Appears in collection : ALCOCRYPT - ALgebraic and combinatorial methods for COding and CRYPTography
APN and AB functions are S-boxes with optimal resistance to the linear and differential cryptanalysis. In this talk we survey known constructions and classifications of these functions and discuss big open problems for the monomial case. Among these problems are the Dobbertin's conjecture on nonexistence of new APN monomials (open since 2000), the Walsh spectrum of Dobbertin's APN monomials (open since 2000), the existence of APN permutations of the form $x^d+L(x)$ where $x^d$ is some of the known APN monomials and $L$ is a nonzero linear map.
Remark : On page 18 the speaker refers the classification result by Brinkmann [3] for functions from the field $F_{2^4}$ of order 16 to itself.