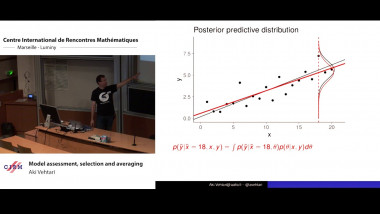
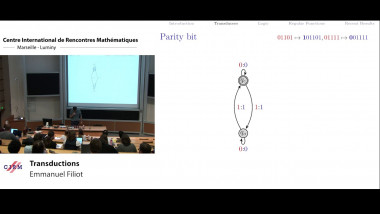
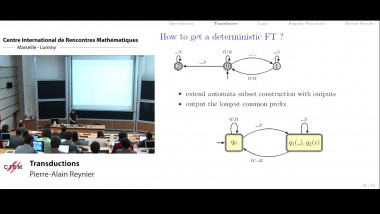
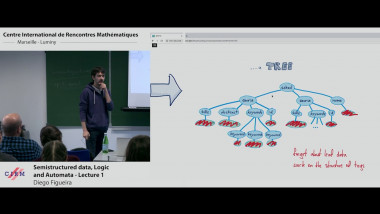
Scales in geophysical flows - Lecture 1
De Rupert Klein
Apparaît également dans la collection : CEMRACS : Geophysical Fluids, Gravity Flows / CEMRACS : Fluides géophysiques, écoulements gravitaires
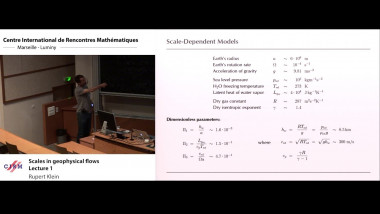
Earth’s atmosphere hosts a rich spectrum of phenomena that involve interactions of a variety of processes across many length and time scales. A systematic approach to analyzing these scale dependent processes is a core task of theoretical meteorology and a prerequi- site to constructing reliable computational models for weather forecasting and climate simulation.
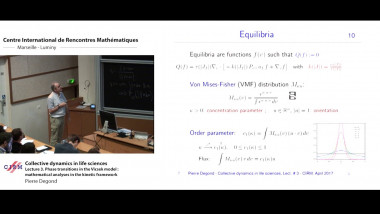
Lecture I The fundamental tools of similarity theory and formal single scale asymptotics will allow us to systematize the large zoo of scale-dependent model equations that one finds in modern textbooks of theoretical meteorology.
Lecture II The meteorological analogue of the incompressible flow equations are the ”anelastic” and ”pseudo-incompressible” models. Here we will learn how the presence of internal gravity waves in the atmosphere implies an asymptotic three-scale problem that renders the formal derivation and justification of these models much more intricate than the classical low Mach number derivation of the incompressible flow equations.
Lecture III The mechanisms by which tropical storms develop into hurricanes and typhoons are still under intense debate despite decades of research. A recent theory for the dynamics of strongly tilted atmospheric vortices will show how asymptotic methods help structuring this scientific debate, and how they offer new angles of scientific attack on the problem.
Lecture ² If time permits, I will also summarize some ramifications of the scaling regimes and scaling theories considered in Lectures I-III on the construction of reliable computational methods.