Mutually enriching connections between ergodic theory and combinatorics - part 5
Apparaît également dans la collection : Jean-Morlet Chair - Doctoral school: Applications of Ergodic Theory in Number Theory / Chaire Jean-Morlet - Ecole doctorale : Applications de la théorie ergodique à la théorie des nombres
² The early results of Ramsey theory : Hilbert's irreducibility theorem, Dickson-Schur work on Fermat's equation over finite fields, van der Waerden's theorem, Ramsey's theoremand its rediscovery by Erdos and Szekeres.
² Three main principles of Ramsey theory : First principle: Complete disorder is impossible. Second principle: Behind every 'Partition' result there is a notion of largeness which is responsible for a 'Density' enhancement of this result. Third principle: The sought-after configurations which are always to be found in large sets are abundant.
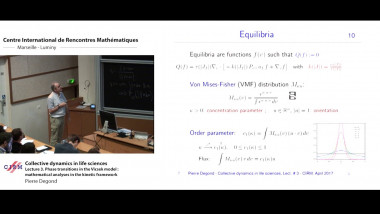
² Furstenberg's Dynamical approach : Partition Ramsey theory and topological dynamics Dynamical versions of van der Waerden's theorem, Hindman's theorem and Graham-Rothschild-Spencer's geometric Ramsey. Density Ramsey theory and Furstenberg's correspondence principle Furstenberg's correspondence principle. Ergodic Szemeredi's theorem. Polynomial Szemeredi theorem. Density version of the Hales-Jewett theorem.
² Stone-Cech compactifications and Hindman's theorem : Topological algebra in Stone-Cech compactifications. Proof of Hind-man's theorem via Poincare recurrence theorem for ultrafilters.
² IP sets and ergodic Ramsey theory : Applications of IP sets and idempotent ultrafilters to ergodic-theoretical multiple recurrence and to density Ramsey theory. IP-polynomial Szemeredi theorem.
² Open problems and conjectures
If time permits: ² The nilpotent connection, ² Ergodic Ramsey theory and amenable groups