Paradigms for the algorithms on different technologies - lecture 1
By Thomas Ayral
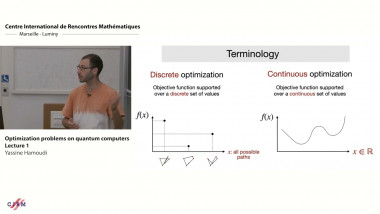
Optimization problem on quantum computers - lecture 1
By Yassine Hamoudi
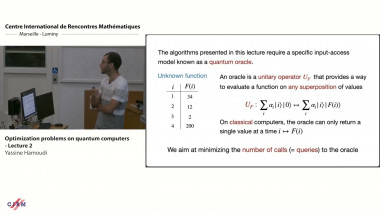
Optimization problem on quantum computers - lecture 2
By Yassine Hamoudi