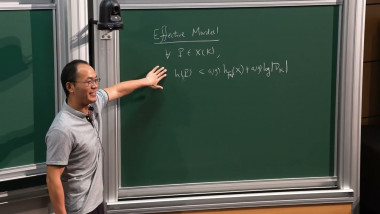
A Quantitative Version of the Uniform Mordell Conjecture
By Xinyi Yuan

Proportionality and the arithmetic volumes of Shimura varieties and the moduli of Shtukas
By Wei Zhang
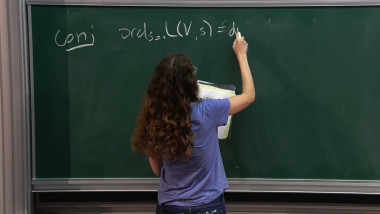
On the Bloch–Kato Conjecture for some four-dimensional symplectic Galois representations
By Naomi Sweeting
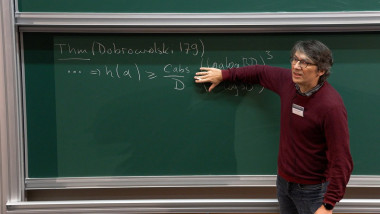
The Dynamical Schinzel-Zassenhaus Conjecture and the Transfinite Diameter of Trees
By Philipp Habegger












