Le transport optimal en pratique : géométrie, algorithmes et applications
By Jean Feydy
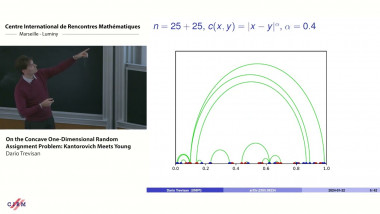
On the concave one-dimensional random assignment problem: Kantorovich meets young
By Dario Trevisan

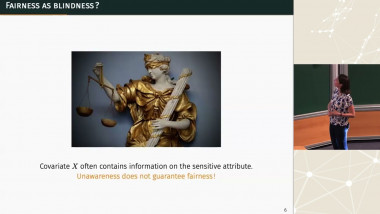
Relationship between classification and regression in statistical fairness
By Solenne Gaucher

Merging rate of opinions via optimal transport on random measures
By Marta Catalano