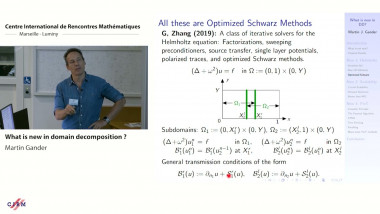
What is new in domain decomposition ?
Domain decomposition research intensified in the early nineties, and there is still substantial research activity in this field. There has been however an important shift in domain decomposition, and I will explain three new interesting research directions that are pursued very actively at the moment, and give newest results: 1. Iterative solvers for time harmonic wave propagation: time harmonic wave propagation problems are very hard to solve by iterative methods. All classical iterative methods, like Krylov methods, multigrid, and also domain decomposition methods, fail for the key model problem, the Helmholtz equation. There are new, highly promising domain decomposition methods for such problems, which I will present, and I will also state precisely under which conditions they can work well, and when they still fail. 2. Coarse space components: domain decomposition analysis has lacked behind multigrid in the precise understanding of the interaction between the domain decomposition smoother and coarse space solver, and all classical domain decomposition solvers need Krylov acceleration to be effective, while multigrid does not. I will present a new spectral analysis of the Schwarz iteration operator, which allows us to achive as an accurate understanding of two level Schwarz methods as the seminal Fourier analysis of multigrid methods. 3. Time parallelization: new computing architectures have too many computing cores to parallelize only in space for evolution problems. I will present time and space-time domain decomposition methods and explain which can be effective for parabolic and hyperbolic problems.