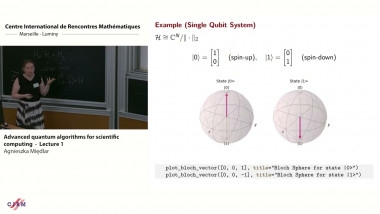
Advanced quantum algorithms for scientific computing - lecture 1
By Agnieszka Międlar

Advanced quantum algorithms for scientific computing - lecture 2
By Agnieszka Międlar

Numerical Simulation and Analysis of Elliptic and Parabolic PDEs using Differential Quadrature Methods and FEMs (1/4)
By JIWARI RAM

Numerical Simulation and Analysis of Elliptic and Parabolic PDEs using Differential Quadrature Methods and FEMs (2/4)
By JIWARI RAM

Numerical Simulation and Analysis of Elliptic and Parabolic PDEs using Differential Quadrature Methods and FEMs (3/4)
By JIWARI RAM











