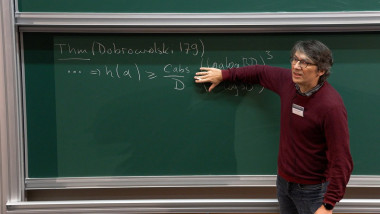1/6 Automorphic Forms and Optimization in Euclidean Space | Vidéo | Carmin.tv

00:00:00 / 00:00:00

Chapters

1/6 Automorphic Forms and Optimization in Euclidean Space

By Maryna Viazovska

Appears in collection : Hadamard Lectures 2019 - Maryna Viazovska - Automorphic Forms and Optimization in Euclidean Space

The goal of this lecture course, “Automorphic Forms and Optimization in Euclidean Space”, is to prove the universal optimality of the $E_8$ and Leech lattices. This theorem is the main result of a recent preprint “Universal Optimality of the $E_8$ and Leech Lattices and Interpolation Formulas”, written in collaboration with H. Cohn, A. Kumar, S.D. Miller and D. Radchenko. We prove that the $E_8$ and Leech lattices minimize energy of every potential function that is a completely monotonic function of squared distance (for example, inverse power laws or Gaussians).

This theorem implies recently proven optimality of $E_8$ and Leech lattices as sphere packings and broadly generalizes it to long-range interactions. The key ingredient of the proof is sharp linear programming bounds. To construct the optimal auxiliary functions attaining these bounds, we prove a new interpolation theorem.

At the last lecture, we will discuss open questions and conjectures which arose from our work.

Information about the video

Date of recording 23/04/2019
Date of publication 23/04/2019
Institution IHES
Licence CC BY-NC-ND
Language English
Audience Researchers, Graduate Students
Director(s) Mandarine Audiovisuel
Format MP4

Domain(s)

Bibliography

H. Cohn, A. Kumar, S. D. Miller, D. Radchenko and M. Viazovska, Universal optimality of the $E_8$ and Leech lattices and interpolation formulas, arXiv:1902.05438

MSC codes

Document(s)

Notes

Last related questions on MathOverflow

You have to connect your Carmin.tv account with mathoverflow to add question

Ask a question on MathOverflow

Copyright Carmin.tv 2024

Give feedback