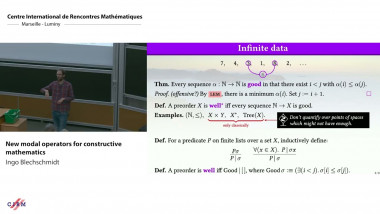
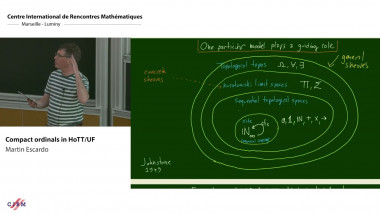
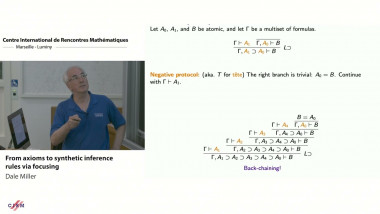
Collection Type Theory, Constructive Mathematics and Geometric Logic / Théorie des types, mathématiques constructives et logique géométrique
The debate between Hilbert and Brouwer, which took place at the beginning of the 20th century, is without doubt one of the most important events in the history of foundations of mathematics. In that debate Brouwer rejected transcendent proof methods and insisted on the exclusive use of intuitionist (or constructive) arguments for a conceptually correct development of mathematics. Hilbert, on the contrary, viewed these transcendental and non-effective proofs as the very essence of mathematical thinking. He introduced proof theory in the hope to justify these transcendent methods by purely effective means. While Gödel’s second incompleteness theorem is generally said to contravene Hilbert’s hopes, it is remarkable that Gödel himself insisted on the fact that his result does not contradict at all Hilbert’s program. In fact, the impact of Gödel’s result on Hilbert’s program was the topic of a discussion between Gödel, Herbrand and Neumann about the fundamental question of the scope of constructive mathematics. Recent results in different fields of mathematics, such as proof theory, type theory, constructive algebra and categorical logic, shed new light on this question. For instance, proof theory seems to indicate precise limits to intuitionistic type theory, while on the other hand the Univalence Axiomallows for a rather unexpected extension of constructive methods. The goal of this workshop is precisely to bring together experts in these different fields to evaluate the impact of those new results on foundations of mathematics.
Organizer(s) Coquand, Thierry ; Negri, Sara ; Rathjen, Michael ; Schuster, Peter
Date(s) 01/05/2023 - 05/05/2023
linked URL https://conferences.cirm-math.fr/2319.html