Operators on analytic function spaces / Opérateurs sur des espaces de fonctions analytiques

Collection Operators on analytic function spaces / Opérateurs sur des espaces de fonctions analytiques

Organizer(s) Fricain, Emmanuel ; Garcia, Stephan Ramon ; Gorkin, Pamela ; Hartmann, Andreas ; Mashreghi, Javad

Date(s) 02/12/2024 - 06/12/2024

linked URL https://conferences.cirm-math.fr/3085.html

00:00:00 / 00:00:00

4 5

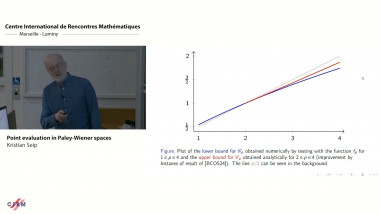
Common range of co-analytic Toeplitz operators on the Drury-Arveson space

By John McCarthy

We shall describe $\cap_m \operatorname{ran} M_m^²$, where $m$ ranges over the cyclic multipliers of the DruryArveson space $H_d^2$, and $M_m$ denotes multiplication by $m$ on $H_d^2$. I will try to convince the audience that there is some interesting functional analysis behind the description.

This is joint work with Alexander Aleman, Michael Hartz and Stefan Richter.

Information about the video

Date of recording 03/12/2024
Date of publication 13/12/2024
Institution CIRM
Licence CC BY NC ND
Language English
Audience Researchers, Graduate Students
Director(s) Luca Recanzone
Format MP4

Citation data

DOI 10.24350/CIRM.V.20273603
Cite this video McCarthy, John (03/12/2024). Common range of co-analytic Toeplitz operators on the Drury-Arveson space. CIRM. Audiovisual resource. DOI: 10.24350/CIRM.V.20273603
URL https://dx.doi.org/10.24350/CIRM.V.20273603

Domain(s)

Bibliography

AGLER, Jim et MCCARTHY, John E. Complete Nevanlinna–Pick kernels. Journal of Functional Analysis, 2000, vol. 175, no 1, p. 111-124. - https://doi.org/10.1006/jfan.2000.3599
AGLER, Jim et MCCARTHY, John E. Pick interpolation and Hilbert function spaces. American Mathematical Society, 2023. - https://doi.org/10.1090/gsm/044
ALEMAN, Alexandru, HARTZ, Michael, MCCARTHY, John E., et al. The Smirnov class for spaces with the complete Pick property. Journal of the London Mathematical Society, 2017, vol. 96, no 1, p. 228-242. - https://doi.org/10.1112/jlms.12060
ALEMAN, Alexandru, HARTZ, Michael, MCCARTHY, John E., et al. Factorizations induced by complete Nevanlinna–Pick factors. Advances in Mathematics, 2018, vol. 335, p. 372-404. - https://doi.org/10.1016/j.aim.2018.07.013
ALEMAN, Alexandru, HARTZ, Michael, MCCARTHY, John E., et al. Multiplier tests and subhomogeneity of multiplier algebras. Documenta Mathematica, 2022, vol. 27, p. 719-764. - https://doi.org/10.48550/arXiv.2008.00981
ARVESON Whilliam .Subalgebras of C²-algebras III: Multivariable operator theory. Acta Math. 181 (2) 159 - 228, 1998 - https://doi.org/10.1007/BF02392585
BORICHEV, Alexander, FRANK, Rupert, et VOLBERG, Alexander. Counting eigenvalues of Schr\" odinger operator with complex fast decreasing potential. arXiv preprint arXiv:1811.05591, 2018. - https://doi.org/10.48550/arXiv.1811.05591
DAVIDSON, Kenneth, RAMSEY, Christopher, et SHALIT, Orr. Operator algebras for analytic varieties. Transactions of the American Mathematical Society, 2015, vol. 367, no 2, p. 1121-1150. - https://doi.org/10.1090/S0002-9947-2014-05888-1
DAVIS, B. Mark et MCCARTHY, John E. Multipliers of de Branges spaces. Michigan Math. J, 1991, vol. 38, no 2, p. 225-240. - https://doi.org/10.1307/mmj/1029004330
EDGAR, G. A. Two function-space topologies. Proceedings of the American Mathematical Society, 1973, vol. 39, no 1, p. 219-220. - https://doi.org/10.1090/S0002-9939-1973-0313771-3
EDWARDS, Robert E. Functional Analysis: Theory and Applications. Dover, New York. 1995.
EL-FALLAH, Omar, KELLAY, Karim, MASHREGHI, Javad, et al. A primer on the Dirichlet space. Cambridge University Press, 2014. - https://doi.org/10.1017/CBO9781107239425
GARNETT, John. Bounded analytic functions.GTM Vol.236 . Springer Science & Business Media, 2006. - https://doi.org/10.1007/0-387-49763-3
HARTZ, Michael. On the isomorphism problem for multiplier algebras of Nevanlinna-Pick spaces. Canadian Journal of Mathematics, 2017, vol. 69, no 1, p. 54-106. - https://doi.org/10.4153/CJM-2015-050-6
HELSON, Henry. Large analytic functions. II. in Analysis and partial differential equations, 1990, vol. 122, p. 217-220.
KORENBLUM, Boris et MCCARTHY, John E. The range of Toeplitz operators on the ball. Revista Matemática Iberoamericana, 1996, vol. 12, p. 47-62. - https://doi.org/10.4171/rmi/194
MCCARTHY, John E. Common range of co-analytic Toeplitz operators. Journal of the American Mathematical Society, 1990, vol. 3, no 4, p. 793-799. - https://doi.org/10.2307/1990902
MCCARTHY, John E. Topologies on the Smirnov class. Journal of functional analysis, 1992, vol. 104, no 1, p. 229-241. - https://doi.org/10.1016/0022-1236(92)90096-2
NAWROCKI, M. Linear functionals on the Smirnov class of the unit ball in Cn. Annales Fennici Mathematici, 1989, vol. 14, no 2, p. 369-379. - https://doi.org/10.5186/aasfm.1989.1421
PAU, Jordi et PELÁEZ, José. On the zeros of functions in Dirichlet-type spaces. Transactions of the American Mathematical Society, 2011, vol. 363, no 4, p. 1981-2002. - https://doi.org/10.1090/S0002-9947-2010-05108-6
PRIWALOW I. I. . Randeigenschaften analytischer Funktionen, Zweite, unter Redaktion von A. I. Markuschewitsch überarbeitete und ergänzte Auflage. Hochschulbücher für Mathematik, Bd. 25, VEB Deutscher Verlag der Wissenschaften, Berlin, 1956.
RUDIN Walter. Function Theory in the unit ball of Cn, Springer-Verlag, Berlin, 1980
RUDIN Walter. New constructions of functions holomorphic in the unit ball of cn, C.B.M.S. No. 63, American Mathematical Society, Providence, 1986.
RUDIN Walter. Functional analysis, Second, International Series in Pure and Applied Mathematics, McGraw-Hill Inc., New York, 1991
SARASON, Donald. Sub-Hardy Hilbert spaces in the unit disk, University of Arkansas Lecture Notes, Wiley, New York, 1994
SHALIT, Orr. (2015). Operator theory and function theory in drury-arveson space and its quotients. In Operator Theory (Vol. 2-2, pp. 1125-1180). Springer Basel. - https://doi.org/10.1007/978-3-0348-0667-1_60
SHAPIRO, Joel H. et SHIELDS, Allen L. Unusual topological properties of the Nevanlinna class. American Journal of Mathematics, 1975, vol. 97, no 4, p. 915-936. - https://doi.org/10.2307/2373681
WILANSKY Albert, Modern methods in topological vector spaces, McGraw-Hill International Book Co., New York, 1978
YANAGIHARA Niro .The containing Fréchet space for the class N +. Duke Math. J. 40 (1) 93 - 103, March 1973 - https://doi.org/10.1215/S0012-7094-73-04010-6
YANAGIHARA, Niro. Multipliers and linear functionals for the class 𝑁⁺. Transactions of the American Mathematical Society, 1973, vol. 180, p. 449-461. - https://doi.org/10.1090/S0002-9947-1973-0338382-X
YANAGIHARA, Niro. Mean growth and Taylor coefficients of some classes of functions. Annales Polonici Mathematici 30 (1). 1974. p. 37-48. - http://eudml.org/doc/263410

Last related questions on MathOverflow

You have to connect your Carmin.tv account with mathoverflow to add question

Ask a question on MathOverflow

All the collection videos

44:52

published on December 13, 2024

Some recent results on generic properties of contractions on Banach spaces

By Sophie Grivaux

39:51

published on December 13, 2024

Point evaluation in Paley–Wiener spaces

By Kristian Seip

37:43

published on December 13, 2024

Linear isometries on the Fréchet space of holomorphic functions on the open unit disc and the annulus

By Isabelle Chalendar

38:06

published on December 13, 2024

Common range of co-analytic Toeplitz operators on the Drury-Arveson space

By John McCarthy

38:09

published on December 13, 2024

von Neumann's inequality on the polydisc

By Michael Hartz

Copyright Carmin.tv 2025

Give feedback