Algebra, arithmetic and combinatorics of differential and difference equations / Algèbre, arithmétique et combinatoire des équations différentielles et aux différences

Collection Algebra, arithmetic and combinatorics of differential and difference equations / Algèbre, arithmétique et combinatoire des équations différentielles et aux différences

Organizer(s) Adamczewski, Boris ; Delaygue, E. ; Raschel, Kilian ; Roques, Julien

Date(s) 28/05/2018 - 01/06/2018

linked URL https://conferences.cirm-math.fr/1761.html

00:00:00 / 00:00:00

2 5

Chapters

Galois theory and walks in the quarter plane

By Charlotte Hardouin

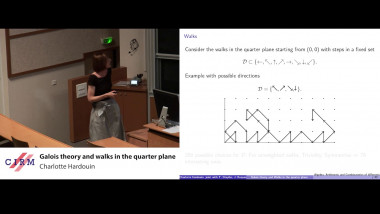
In the recent years, the nature of the generating series of walks in the quarter plane has attracted the attention of many authors in combinatorics and probability. The main questions are: are they algebraic, holonomic (solutions of linear differential equations) or at least hyperalgebraic (solutions of algebraic differential equations)? In this talk, we will show how the nature of the generating function can be approached via the study of a discrete functional equation over a curve E, of genus zero or one. In the first case, the functional equation corresponds to a so called q-difference equation and all the related generating series are differentially transcendental. For the genus one case, the dynamic of the functional equation corresponds to the addition by a given point P of the elliptic curve E. In that situation, one can relate the nature of the generating series to the fact that the point P is of torsion or not.

Information about the video

Date of recording 30/05/2018
Date of publication 06/06/2018
Institution CIRM
Licence CC BY NC ND
Language English
Director(s) Guillaume Hennenfent
Format MP4

Citation data

DOI 10.24350/CIRM.V.19409503
Cite this video Hardouin, Charlotte (30/05/2018). Galois theory and walks in the quarter plane. CIRM. Audiovisual resource. DOI: 10.24350/CIRM.V.19409503
URL https://dx.doi.org/10.24350/CIRM.V.19409503

Domain(s)

Bibliography

Dreyfus, T., Hardouin, C., Roques, J., & Singer, M.F. (2018). On the nature of the generating series of walks in the quarter plane. Inventiones mathematicae - https://doi.org/10.1007/s00222-018-0787-z
Dreyfus, T., Hardouin, C., Roques, J., & Singer, M.F. (2017). Walks in the quarter plane, genus zero case. <arXiv:1710.02848> - https://arxiv.org/abs/1710.02848

MSC codes

Last related questions on MathOverflow

You have to connect your Carmin.tv account with mathoverflow to add question

Ask a question on MathOverflow

All the collection videos

52:52

published on May 31, 2018

A supercongruence and hypergeometric motives

By Frits Beukers

49:26

published on June 6, 2018

Galois theory and walks in the quarter plane

By Charlotte Hardouin

54:38

published on May 31, 2018

Around Jouanolou-type theorems

By Rahim Moosa

53:45

published on May 31, 2018

Gamma functions, monodromy and Apéry constants

By Masha Vlasenko

57:05

published on June 4, 2018

The classification of excursions

By Marni Mishna

Copyright Carmin.tv 2024

Give feedback