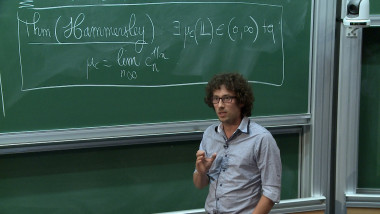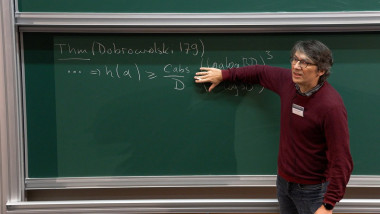Large gaps between primes in subsets
Apparaît également dans les collections : Prime numbers and automatic sequences: determinism and randomness / Nombres premiers et suites automatiques : aléa et déterminisme, The Fields Medallists
All previous methods of showing the existence of large gaps between primes have relied on the fact that smooth numbers are unusually sparse. This feature of the argument does not seem to generalise to showing large gaps between primes in subsets, such as values of a polynomial. We will talk about recent work which allows us to show large gaps between primes without relying on smooth number estimates. This then generalizes naturally to show long strings of consecutive composite values of a polynomial. This is joint work with Ford, Konyagin, Pomerance and Tao.