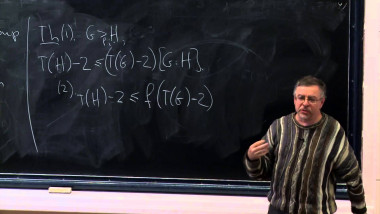Carmin.tv

Mathématiques
et Interactions

Merci de nous donner votre avis pour que nous puissions améliorer votre expérience !
Not Only Scalar Curvature Seminar
74 videos

Not Only Scalar Curvature Seminar

Geometric structures and discrete group actions / Structures géométriques et actions de groupes discrets
4 videos

Geometric structures and discrete group actions / Structures géométriques et actions de groupes discrets

Misha Gromov : Mathematical Description of Biological Structures
4 videos

Misha Gromov : Mathematical Description of Biological Structures

Applications of NonCommutative Geometry to Gauge Theories, Field Theories, and Quantum Space-Time / Applications de la Géométrie Non Commutative aux Théories de Jauge, à la Théorie des Champs et aux Espaces-Temps Quantiques
5 videos

Applications of NonCommutative Geometry to Gauge Theories, Field Theories, and Quantum Space-Time / Applications de la Géométrie Non Commutative aux Théories de Jauge, à la Théorie des Champs et aux Espaces-Temps Quantiques

Toutes les collections
CIMPA
CIRM
IHES
IHP
LMR
SMF
Tous les instituts
2014 - T1 - WS1 - Asymptotic properties of groups

Collection 2014 - T1 - WS1 - Asymptotic properties of groups

Organisateur(s) Breuillard, Emmanuel ; Chatterji, Indira ; Erschler, Anna
Date(s) 24/03/2014 - 28/03/2014
URL associée https://web.archive.org/web/20220123001204/https://sites.google.com/site/geowalks2014/home/workshop
00:00:00 / 00:00:00
8 21

Imbeddings in groups of subexponential growth

De Laurent Bartholdi

A finitely generated group has subexponential growth if the number of group elements expressible as words of length $\le n$ growssubexponentially in $n$. I will show that every countable group that does not contain asubgroup of exponential growth imbeds in a finitely generated group ofsubexponential growth. This shows that there are no restrictions on being a subgroup of a group of exponential growth, except the obvious ones. This produces in particular the first examples of groups ofsubexponential growth containing $\mathbb Q$. This also producesgroups of subexponential growth and arbitrarily large distortion in uniformly convex Banach (e. g. \ Hilbert) spaces. This is joint work with Anna Erschler.

Informations sur la vidéo

  • Date de publication 14/04/2014
  • Institut IHP
  • Licence CC BY-NC-ND
  • Format MP4

Domaine(s)

Codes MSC

Dernières questions liées sur MathOverflow

Pour poser une question, votre compte Carmin.tv doit être connecté à mathoverflow

Poser une question sur MathOverflow




Inscrivez-vous

  • Mettez des vidéos en favori
  • Ajoutez des vidéos à regarder plus tard &
    conservez votre historique de consultation
  • Commentez avec la communauté
    scientifique
  • Recevez des notifications de mise à jour
    de vos sujets favoris
Copyright Carmin.tv 2025
En poursuivant votre navigation sur ce site, vous acceptez l'utilisation de cookies pour améliorer votre expérience. En savoir plus sur notre politique de confidentialité.
Accepter
Donner son avis