56:53
published on April 18, 2025
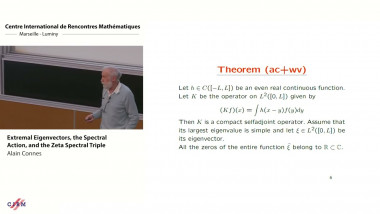
Extremal eigenvectors, the spectral action, and the zeta spectral triple
By Alain Connes
Topos and Noncommutative Geometry: Two Perspectives on Space and Numbers
By Alain Connes
Appears in collection : 2025 - T1 - WS2 - Tempered representations and K-theory
Noncommutative geometry and the notion of topos are two mathematical concepts that provide complementary perspectives on the structure of a space. In this talk, I will begin by explaining, as simply as possible, these two concepts and what makes them unique. The originality of noncommutative geometry can be directly perceived through the existence of an intrinsic time evolution of a noncommutative space. The originality of toposes can similarly be perceived through the intuitionistic logic associated with a topos. It is the metric structure, embodied by a representation—as operators in Hilbert space—of coordinates and the length element, that allows noncommutative geometry to engage with reality, namely the structure of space-time at the infinitesimally small scale as revealed by contemporary physics through the Standard Model. As for toposes, it is the additional structure of a sheaf of algebras that enables geometry to manifest beyond topology.
In the second part of the talk, I will explain how the spectrum of the ring of integers can be understood through these two geometric lenses. The connection between these two approaches rests on an extension of class field theory that sheds light on the analogy established by Mumford and Mazur between knots and prime numbers. The spectral perception of the ring of integers naturally emerges from the study of the zeros of the Riemann zeta function, thereby revealing deep structures at the interface of arithmetic, topology, and geometry.
