
01:39:42
published on July 4, 2025
Lecture 3: What is the Universal Scaling Limit of Random Interface Growth, and What Does It Tell Us?
By Ivan Corwin
01:35:26
published on July 4, 2025
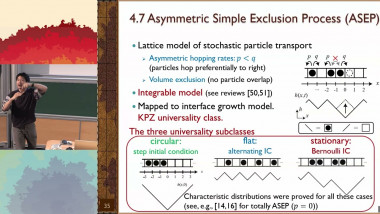
Introduction to the Physics of the KPZ Universality Class (3/3)
By Kazumasa Takeuchi












