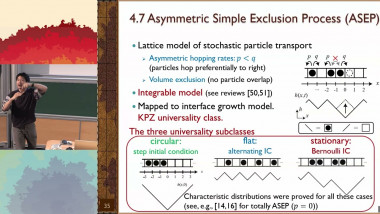
Introduction to the Physics of the KPZ Universality Class (3/3)
By Kazumasa Takeuchi
Nonequilibrium Point Processes with Long-range Correlations Generated by Stochastic Resetting (3/3)
By Satya N. Majumdar
Appears in collection : Combinatorics and Arithmetic for Physics : Special Days
Using the matrix-forest theorem and the Parisi-Sourlas trick we formulate and solve a one-matrix model with non-polynomial potential which provides perturbation theory for massive spinless fermions on dynamical planar graphs. This is a version of 2d quantum gravity discretized via RRG coupled to massive spinless fermions. Our model equivalently describes the ensemble of spanning forests on the same graph. The solution is formulated in terms of an elliptic curve. We then focus on a near-critical scaling limit when both the graphs and the trees in the forests are macroscopically large. In this limit we obtain universal one-point scaling functions (condensates), parameterized in terms of the Lambert function. Our results provide a rare example where one can explore the flow between two gravity models – in this case, the theories of conformal matter coupled to 2d gravity with c=-2 (large trees regime) and c=0 (small trees regime). We shall also present the results of numerical simulations concerning phase transitions in RRG ensemble and their relation with Anderson localization.
