published on December 7, 2023
A new proof of Viazovska's modular form inequalities for sphere packing in dimension 8
By Dan Romik
Sobolev spaces on metric spaces
By Jun Kigami
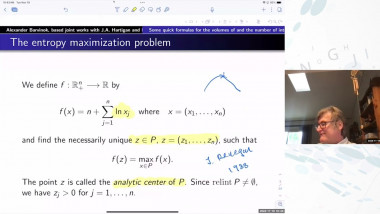
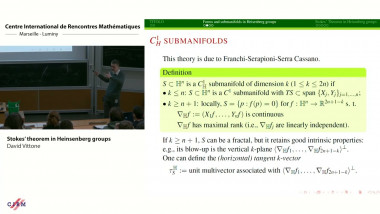
Appears in collection : Analysis on fractals and networks, and applications / Analyse sur les fractals et les réseaux, et applications
Traditionally, theories of “Sobolev” spaces on metric spaces have used local Lipschitz constants as a substitute for the gradient of functions. However, a recent study by Kajino and Murugan revealed that such an idea does not work for a class of self-similar sets including the planar Sierpinski carpet. The notion of conductive homogeneity was proposed to construct a counterpart of Sobolev spaces and Sobolev p-energy even for such cases. In this talk, I will review the method of construction of Sobolev spaces under the conductive homogeneity and give a class of regular polygon-based self-similar sets having the conductive homogeneity. Our condition is the local symmetry of the space with some (or no) global symmetry. In particular, we show that any locally symmetric triangle-based self-similar sets possess the conductive homogeneity. This is joint work with Y. Ota.
