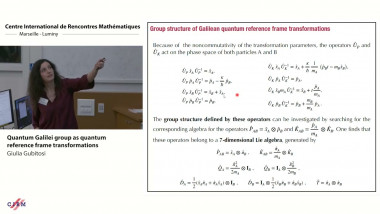
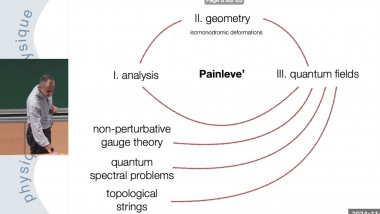
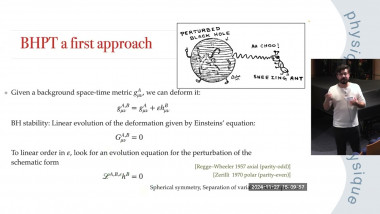
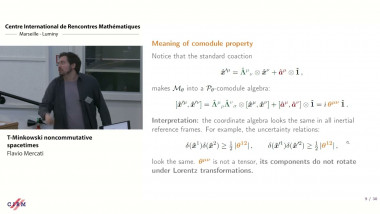
Appears in collection : 2024 - PC1 - WS - Curved spacetimes, field theory and beyond

Null infinity, $\mathcal{I}^+$, arises as a boundary of the Penrose conformal completion $(\hat{M}, \hat{g}_{ab})$ of an asymptotically flat physical space-time $(M, g_{ab})$. I will begin by pointing out that $\mathcal{I}^+$ is a weakly isolated horizon (WIH) in $(\hat{M}, \hat{g}_{ab})$, and then show that all its familiar properties can be derived from the general WIH framework. This seems quite surprising because physics associated with black hole (and cosmological) WIHs $\Delta$ is very different from that extracted at $\mathcal{I}^+$. These differences can be directly traced back to the fact that while Einstein's equations hold at $\Delta$, conformal Einstein's equations hold at $\mathcal{I}^+$. In particular, the BMS group at $\mathcal{I}^+$ stems from the symmetry group of WIHs. There is also a unified procedure to arrive at fluxes and charges associated with the BMS symmetries at $\mathcal{I}^+$ and those associated with the symmetries at $\Delta$. This procedure differs from those commonly used in the literature and its novel elements seem interesting in their own right. This subtle interplay between geometry and physics is reminiscent of a musical fugue.

The fact that is there is a single mathematical framework underlying $\Delta$ and $\mathcal{I}^+$ paves the way to explore the relation between horizon dynamics in the strong field region and waveforms at infinity. It should also be useful in the analysis of black hole evaporation in quantum gravity. This work was carried out in collaboration with Simone Speziale and supported by Eberly and Atherton Funds of Penn State and the DVRC program of the Perimeter Institute.