
Strongly ergodic equivalence relations and full factors
By Amine Marrakchi
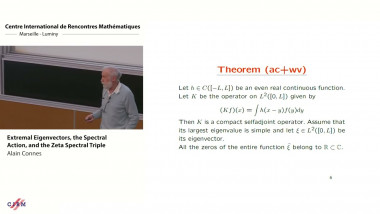
Extremal eigenvectors, the spectral action, and the zeta spectral triple
By Alain Connes

Resonances and residue operators for pseudo-Riemannian hyperbolic spaces
By Polyxeni Spilioti

C-algebras for real reductive symmetric spaces and K-theory
By Shintaro Nishikawa











