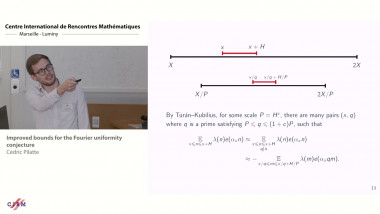
Construction of $p$-adic $L$-functions for unitary groups
Appears in collection : Arithmetic geometry, representation theory and applications / Géométrie arithmétique, théorie des représentations et applications
This is a report on the construction of $p$-adic $L$-functions attached to ordinary families of holomorphic modular forms on the unitary groups of $n$-dimensional hermitian vector spaces over $CM$ fields. The results have been obtained over a period of nearly 15 years in joint work with Ellen Eischen, Jian-Shu Li, and Chris Skinner. The $p$-adic $L$-functions specialize at classical points to critical values of standard $L$-functions of cohomological automorphic forms on unitary groups, or equivalently of cohomological automorphic forms on $GL(n)$ that satisfy a polarization condition. When $n = 1$ one recovers Katz's construction of $p$-adic $L$-functions of Hecke characters.