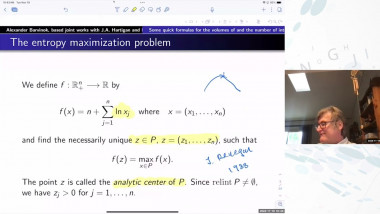
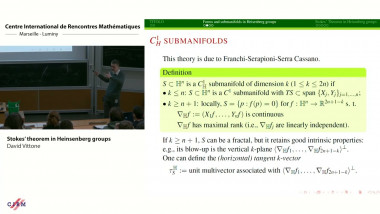
Appears in collection : 2023 - T3 - WS3 - Computer algebra for functional equations in combinatorics and physics

Maryna Viazovska in 2016 found a remarkable application of the theory of modular forms to a fundamental problem in geometry, obtaining a solution to the sphere packing problem in dimension 8 through an explicit construction of a so-called "magic function" that she defined in terms of classical functions, the Eisenstein series and Jacobi thetanull functions. The same method also led shortly afterwards to the solution of the sphere packing in dimension 24 by her and several collaborators. One component of Viazovska's proof consisted of proving a pair of inequalities satisfied by the modular forms she constructed. Viazovska gave a proof of these inequalities that relied in an essential way on computer calculations. In this talk I will present a new proof of Viazovska's inequalities that uses only elementary arguments that can be easily checked by a human.