Collection Yifeng Liu - Derivative of L-functions for unitary groups
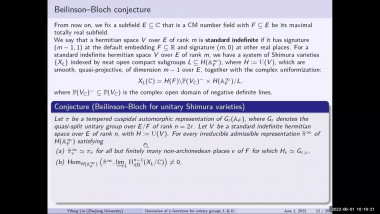
In this lecture series, we will focus on the recent advance on the Beilinson-Bloch conjecture for unitary Shimura varieties, more precisely, a Gross-Zagier type formula for automorphic forms on unitary groups of higher ranks. We will start from the general theory of height pairings between cycles on varieties over number fields. Then we will introduce the doubling method, a powerful tool in the theory of automorphic forms for studying L-functions for classical groups, in particular, unitary groups. Finally, we will combine the automorphic side and the arithmetic side to outline the proof of a formula relating the central derivative of L-function and the height of special cycles on Shimura varieties.
Appears in collection : Franco-Asian Summer School on Arithmetic Geometry
Organizer(s) Ahmed Abbes (CNRS & IHÉS), Ana Caraiani (Imperial College London ), Ariane Mézard (Sorbonne Université), Takeshi Saito (University of Tokyo), Takeshi Tsuji (The University of Tokyo), Daxin Xu (Chinese Academy of Sciences), Weizhe Zheng (Chinese Academy of Sciences).
Date(s) 01/06/2022 - 02/06/2022
linked URL https://www.ihes.fr/~abbes/Luminy/luminy2022.html