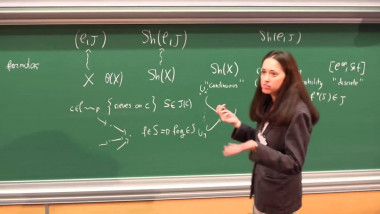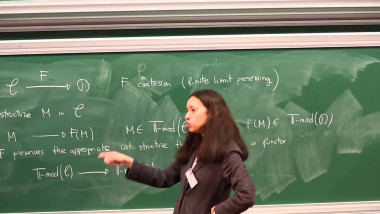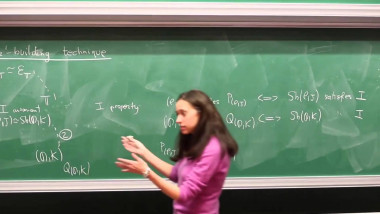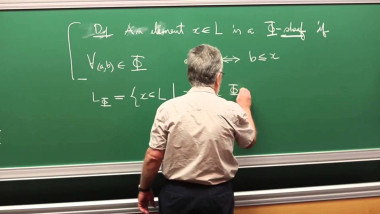Collection Topos à l'IHES
Organizer(s)
Date(s)
30/04/2024
From Essential Inclusions to Local Geometric Morphisms
It is well known that, given a site of denition, a subtopos of Grothendieck topos can be obtained by strengthening the Grothendieck topology, thus obtaining an inclusion of toposes. An essential inclusion is one where the inverse image functor of this inclusion has a left adjoint. Kelly and Lawvere proved in [1] that an inclusion is essential if, and only if, the stronger topology is closed under arbitrary intersections. They also showed that such a topology generates idempotent ideals on the base category of the site, and this fact fully characterises the Grothendieck topologies which give rise to essential inclusions into presheaf toposes. In SGA 4, Grothendieck and Verdier dened a local topos as one where the canonical geometric morphism into Set has an extra right adjoint. One can generalise this denition by taking an arbitrary topos instead of Set, and thus dening a local geometric morphism between two toposes. Such a geometric morphism is always connected, i.e. that the extra adjoint is full and faithful, and this implies that the codomain is a subtopos of the domain. Thus one can view a local geometric morphism as an essential inclusion where the extra left adjoint preserves finite limits, in other words, a cartesian essential inclusion. Somewhat midway between local geometric morphisms and essential inclusions are finite-product-preserving essential inclusions, which are essential inclusions where the leftmost adjoint preserves nite products. The process of understanding the invariant of a topos in terms of its sites of denition is paramount in the "toposes as bridges" approach of Caramello, which is outlined in [2]. In this talk I shall explain how to obtain characterisations of both the sites that induce cartesian essential inclusions and the sites that induce finite-product-preserving essential inclusions, and also offer extensions of the theorem of Kelly and Lawvere, which states that there is a bijection between essential inclusions into a presheaf topos [Cop; Set] and two-sided idem-potent ideals on C. References: 1. G.M. Kelly, F.W. Lawvere - On The Complete Lattice Of Essential Localizations. Bull. Soc. Math. Belg. Ser. A, XLI(2): 289-319, 1989 2. O. Caramello - The Unication of Mathematics Via Topos Theory. arXiv:1006.3930v1 - June 20, 2010.
