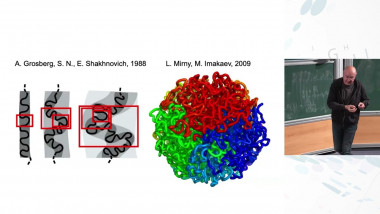
We investigate statistical and topological properties of fractal Brownian motion with short-range interactions. The attention is paid to statistical properties of conformations with the fractal dimension Df ≥ 2 in the three-dimensional space. Using a combination of analytic arguments and Monte Carlo simulations we show that, with the increase of the fractal dimension, Df >2, typical conformations become less knotted. Our study is motivated by an attempt to mimic the statistics of unknotted polymer rings, which are known to equilibrate into the compact hierarchical structure with Df = 3 at large scales. Replacing topologically stabilized conformation by a path with the fractal dimension Df = 3, we tremendously simplify the problem since we wash out the topological constraints from the consideration.
By Leonid Pastur
By Vadim Kaimanovich
By Tatiana Nagnibeda
By Mikhail Gromov
By Andrey Malyutin
By Anna Ershler
By Fyodor Petrov
By Alexander Chervov
By Vladimir Rubtsov
By Alexander Barvinok
By Barbara Schapira
By Barbara Schapira
