Nonlinear Waves Trimester - May Conference

Collection Nonlinear Waves Trimester - May Conference

Organizer(s) Frank MERLE (Université de Cergy-Pontoise & IHÉS), Pierre RAPHAËL (Université Nice Sophia-Antipolis), Nikolay TZVETKOV (Université de Cergy-Pontoise)

Date(s) 23/05/2016 - 27/05/2016

linked URL https://indico.math.cnrs.fr/event/889/

00:00:00 / 00:00:00

12 21

Some Landau damping results for the HMF model and its discrete time approximation

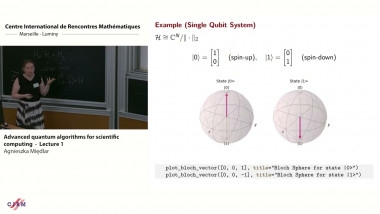
We consider solutions of the Vlasov-HMF model starting in a small Sobolev neighborhood of a spatially homogeneous stationary state satisfying a linear stability criterion and prove a scattering result (Landau damping). We then consider time discretizations of these solutions based on splitting methods between the linear and non-linear part of the equation and we prove that the numerical solutions converge weakly to a modified state which is close to the continuous one. We also prove that our numerical scheme is uniformly convergent, with a convergence rate of order one for Lie splittings, and two for Strang splittings. We will also consider the case of non-homegeous states for which action-angle variables can be used

Information about the video

Date of recording 25/05/2016
Date of publication 03/06/2016
Institution IHES
Licence CC BY-NC-ND
Format MP4

Domain(s)

Numerical Analysis

MSC codes

Last related questions on MathOverflow

You have to connect your Carmin.tv account with mathoverflow to add question

Ask a question on MathOverflow

All the collection videos

40:01

published on June 3, 2016

Wellposedness and scattering for the Zakharov system in four dimensions

By Kenji Nakanishi

55:51

published on June 3, 2016

The Einstein Equations and Gravitational Radiation

By Lydia Bieri

52:06

published on June 3, 2016

Necklace solitary waves on bounded domains

By Gadi Fibich

48:53

published on June 3, 2016

Modified scattering for the Gross-Pitaevskii equation

By Laurent Thomann

59:56

published on June 3, 2016

Bubbling blow - up in critical parabolic problems

By Manuel del Pino

57:27

published on June 3, 2016

Sharp local decay estimates for the Ricci flow on surfaces

By Peter Topping

54:42

published on June 3, 2016

Leapfrogging vortices for the Gross-Pitaevskii equation

By Didier Smets

57:29

published on June 3, 2016

Almost global solutions for the capillarity wave equation with small periodic data

By Jean Marc Delort

01:04:59

published on June 3, 2016

Symplectic non-squeezing for the cubic NLS on the plane

By Monica VISAN

53:33

published on June 3, 2016

Blowup for supercritical equivariant wave maps

By Piotr Bizon

53:21

published on June 3, 2016

Remarks about the self - similar solutions of the Vortex Filament Equation

By Luis Vega

56:30

published on June 3, 2016

Some Landau damping results for the HMF model and its discrete time approximation

By Erwann Faou

55:14

published on June 3, 2016

Quasi - periodic standing wave solutions of gravity-capillary water waves

By Massimiliano Berti

57:01

published on June 3, 2016

Nonlinear stability of expanding stars in the mass-critical Euler-Poisson system

By Mahir Hadzic

46:33

published on June 3, 2016

Stability of line solitons for the KP-II equation

By Tetsu Mizumachi

55:06

published on June 3, 2016

Global regularity and scattering for energy critical geometric wave equations

By Sung Jin Oh

42:15

published on June 3, 2016

Equivariant wave maps on a class of rotationally symmetric manifolds

By Piero D'Ancona

55:43

published on June 3, 2016

A continuous family of energies for the Korteweg - de Vries equation and the cubic nonlinear Schrödinger equation

By Herbert Koch

46:25

published on June 3, 2016

On special regularity properties of a class of dispersive equations

By Gustavo Ponce

53:14

published on June 3, 2016

Birkhoff normal form for nonlinear wave equations

By Walter Craig

59:18

published on June 3, 2016

The problem of stabiliy of Kerr under axilly symmetric perturbations

By Sergiu Klainerman

Copyright Carmin.tv 2025

Give feedback