Chapters
Information about the video
- Date of recording 14/08/2014
- Date of publication 22/08/2014
- Institution CIRM
- Licence CC BY NC ND
- Language English
- Director(s) Guillaume Hennenfent
- Format MP4
Citation data
- DOI 10.24350/CIRM.V.18556703
- Cite this video Ratnani, Ahmed (14/08/2014). Towards complex and realistic tokamaks geometries in computational plasma physics. CIRM. Audiovisual resource. DOI: 10.24350/CIRM.V.18556703
- URL https://dx.doi.org/10.24350/CIRM.V.18556703
Domain(s)
Bibliography
- Ratnani, A. et al. Gasus : Python for IsoGeometric Analysis simulations in Plasmas Physics. (In preparation)
- Ratnani, A. et al. Alignement and equidistribution for two-dimensional grid adaptation using B-splines. (In preparation)
- Ratnani, A. et al. Application of the IsoGeometric mesh adaptation for solving the Anistropic Diffusion problem. (In preparation)
- Baines, M.J. Least squares and approximate equidistribution in multidimensions. Numerical Methods for Partial Dierential Equations, vol. 15 (1999), no. 5, pp. 605-615 - http://dx.doi.org/10.1002/(sici)1098-2426(199909)15:5<605::aid-num7>3.0.co;2-9
- Benamou, J.D., Froese, B. D. and Oberman, A. M. Two numerical methods for the elliptic monge-ampère equation. ESAIM : Mathematical Modelling and Numerical Analysis, vol. 44 (2010), no. 4, pp. 737-758 - http://dx.doi.org/10.1051/m2an/2010017
- Brenier, Y. Polar factorization and monotone rearrangement of vector-valued functions. Communications on Pure and Applied Mathematics, vol. 44 (1991), no. 4, pp. 375-417 - http://dx.doi.org/10.1002/cpa.3160440402
- Budd, C.J., Cullen, M.J.P. and Walsh, E.J. Monge-ampère based moving mesh methods for numerical weather prediction, with applications to the eady problem. Journal of Computational Physics, vol. 236 (2013), pp. 247-270 - http://dx.doi.org/10.1016/j.jcp.2012.11.014
- Delzanno, G.L., Chacon, L., Finn, J.M., Chung, Y. and Lapenta, G. An optimal robust equidistribution method for two-dimensional grid adaptation based on monge-kantorovich optimization. Journal of Computational Physics, vol. 227 (2008), no. 23, pp. 9841-9864 - http://dx.doi.org/10.1016/j.jcp.2008.07.020
- Fasshauer, G.E. and Schumaker, Larry L. Minimal energy surfaces using parametric splines. Computer Aided Geometric Design, vol. 13 (1996), no. 1, pp. 45-79 - http://dx.doi.org/10.1016/0167-8396(95)00006-2
- Floater, M.S. and Hormann, K. Surface parameterization : a tutorial and survey. In Dodgson, N.A. (ed.) et al., Advances in Multiresolution for Geometric Modelling. Mathematics and Visualization. Berlin, Springer, 2005, pp. 157-186. ISBN 3-540-21462-3 - http://dx.doi.org/10.1007/3-540-26808-1_9
- Huang, W. and Russell, R.D. Adaptive moving mesh methods. Applied mathematical sciences, 174. New York, Springer, 2011. xvii, 432 p. ISBN 978-1-4419-7915-5 - http://dx.doi.org/10.1007/978-1-4419-7916-2
MSC codes
Last related questions on MathOverflow
You have to connect your Carmin.tv account with mathoverflow to add questionAsk a question on MathOverflow
-
All the collection videos
![Fluid and transport modeling of plasmas - Lecture 1: collisional plasma kinetics, solutions]()
 01:52:16published on August 25, 2014
01:52:16published on August 25, 2014Fluid and transport modeling of plasmas - Lecture 1: collisional plasma kinetics, solutions
By James D. Callen
![Fluid and transport modeling of plasmas - Lecture 2: kinetic and fluid solutions of PKE]()
 01:49:34published on August 25, 2014
01:49:34published on August 25, 2014Fluid and transport modeling of plasmas - Lecture 2: kinetic and fluid solutions of PKE
By James D. Callen
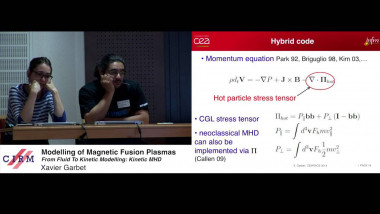
![Modelling of magnetic fusion plasmas: from fluid to kinetic description: kinetic MHD]()
 01:35:03published on August 1, 2014
01:35:03published on August 1, 2014Modelling of magnetic fusion plasmas: from fluid to kinetic description: kinetic MHD
By Xavier Garbet
![Simulation of kinetik electrostatic electron nonlinear (KEEN) waves with variable velocity resolution grids and high-order time-splitting]()
 54:42published on August 25, 2014
54:42published on August 25, 2014Simulation of kinetik electrostatic electron nonlinear (KEEN) waves with variable velocity resolution grids and high-order time-splitting
By Michel Mehrenberger
![Mathematical properties of hierarchies of reduced MHD models]()
 01:03:04published on August 26, 2014
01:03:04published on August 26, 2014Mathematical properties of hierarchies of reduced MHD models
By Bruno Després
![Exact conservation laws for gyrokinetic Vlasov-Poisson equations]()
 55:54published on August 17, 2014
55:54published on August 17, 2014Exact conservation laws for gyrokinetic Vlasov-Poisson equations
By Natalia Tronko
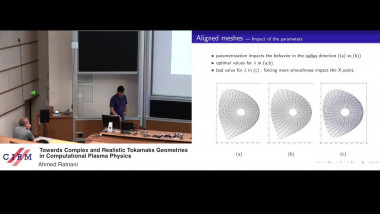
![Towards complex and realistic tokamaks geometries in computational plasma physics]()
 55:29published on August 22, 2014
55:29published on August 22, 2014Towards complex and realistic tokamaks geometries in computational plasma physics
By Ahmed Ratnani
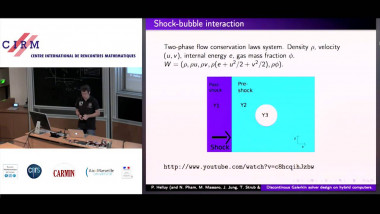
![A simple HLLC-type Riemann solver for compressible non-equilibrium two-phase flows]()
 45:05published on August 26, 2014
45:05published on August 26, 2014A simple HLLC-type Riemann solver for compressible non-equilibrium two-phase flows
By Damien Furfaro
![On hybrid method for rariefied gas dynamics : Boltzmann vs. Navier-Stokes models]()
 59:48published on August 25, 2014
59:48published on August 25, 2014On hybrid method for rariefied gas dynamics : Boltzmann vs. Navier-Stokes models
By Francis Filbet
![Fluid and transport modeling of plasmas - Lecture 3: fluid models for tokamak plasmas]()
 02:00:20published on August 25, 2014
02:00:20published on August 25, 2014Fluid and transport modeling of plasmas - Lecture 3: fluid models for tokamak plasmas
By James D. Callen
![Fluid and transport modeling of plasmas - Lecture 4: tokamak plasma transport modeling]()
 01:59:13published on August 25, 2014
01:59:13published on August 25, 2014Fluid and transport modeling of plasmas - Lecture 4: tokamak plasma transport modeling
By James D. Callen
![Discontinuous Galerkin solver design on hybrid computers]()
 55:47published on August 25, 2014
55:47published on August 25, 2014Discontinuous Galerkin solver design on hybrid computers
By Philippe Helluy
Register
- Bookmark videos
- Add videos to see later &
keep your browsing history - Comment with the scientific
community - Get notification updates
for your favorite subjects