Equivariant formality and reduction | Vidéo | Carmin.tv

00:00:00 / 00:00:00

Equivariant formality and reduction

De Chiara Esposito

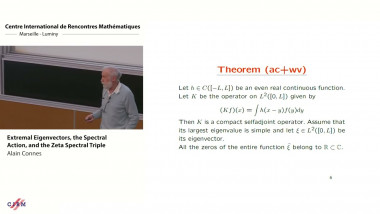
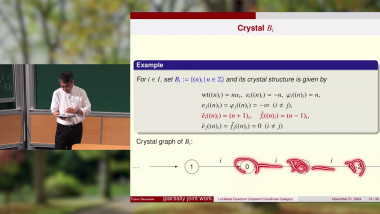
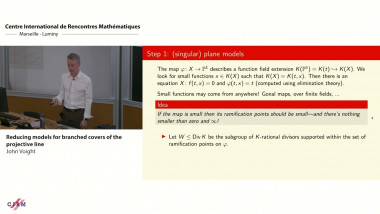
Apparaît dans la collection : Higher Algebra, Geometry, and Topology / Algèbre, Géométrie et Topologie Supérieures

In this talk, we discuss the reduction-quantization diagram in terms of formality. First, we propose a reduction scheme for multivector fields and multidifferential operators, phrased in terms of L-infinity morphisms. This requires the introduction of equivariant multivector fields and equivariant multidifferential operator complexes, which encode the information of the Hamiltonian action, i.e., a G-invariant Poisson structure allowing for a momentum map. As a second step, we discuss an equivariant version of the formality theorem, conjecturedby Tsygan and recently solved in a joint work with Nest, Schnitzer, and Tsygan. This result has immediate consequences in deformation quantization, since it allows for obtaining a quantum moment map from a classical momentum map with respect to a G-invariant Poisson structure.

Informations sur la vidéo

Date de captation 09/05/2024
Date de publication 29/05/2024
Institut CIRM
Licence CC BY NC ND
Langue Anglais
Audience Chercheurs, Doctorants
Réalisateur(s) Guillaume Hennenfent
Format MP4

Données de citation

DOI 10.24350/CIRM.V.20173903
Citer cette vidéo Esposito, Chiara (09/05/2024). Equivariant formality and reduction. CIRM. Audiovisual resource. DOI: 10.24350/CIRM.V.20173903
URL https://dx.doi.org/10.24350/CIRM.V.20173903

Domaine(s)

Algèbres quantiques

Bibliographie

ESPOSITO, Chiara, KRAFT, Andreas, et SCHNITZER, Jonas. The strong homotopy structure of BRST reduction. Pacific Journal of Mathematics, 2023, vol. 325, no 1, p. 47-83. - https://doi.org/10.2140/pjm.2023.325.47
ESPOSITO, Chiara, KRAFT, Andreas, et SCHNITZER, Jonas. The strong homotopy structure of Poisson reduction. Journal of Noncommutative Geometry, 2022, vol. 16, no 3. - https://doi.org/10.4171/JNCG/455

Codes MSC

Dernières questions liées sur MathOverflow

Pour poser une question, votre compte Carmin.tv doit être connecté à mathoverflow

Poser une question sur MathOverflow

Copyright Carmin.tv 2025

Donner son avis